Page 153 - classs 6 a_Neat

11. Copy and draw the reflection of the points, line and triangle in the mirror line ‘ l ’shown below
Two - Dimensional
10 Refelection Symmetry

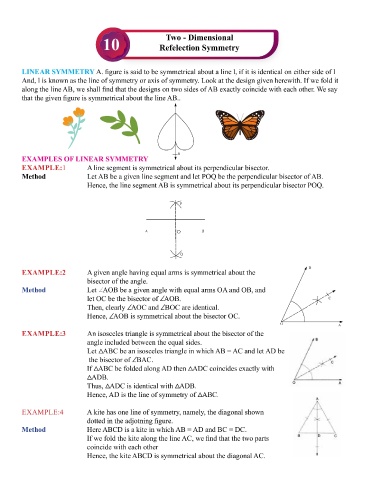
LINEAR SYMMETRY A. figure is said to be symmetrical about a line l, if it is identical on either side of l
And, l is known as the line of symmetry or axis of symmetry. Look at the design given herewith. If we fold it
along the line AB, we shall find that the designs on two sides of AB exactly coincide with each other. We say
that the given figure is symmetrical about the line AB..

12. Which of the digits from 0 to 9 have lines of symmetry? Draw them.
13. Draw five letters of the English alphabet that have one line of symmetry. B
14. Draw the following geometrical shapes and draw a line of symmetry in each of them. EXAMPLES OF LINEAR SYMMETRY
a. Square b. Rectangle c. Hexagon d. Circle e. Kite EXAMPLE:1 A line segment is symmetrical about its perpendicular bisector.
15. Using a ruler and a compass, draw a line segment AB = 5.5 cm. Construct the prependicular bisector of the Method Let AB be a given line segment and let POQ be the perpendicular bisector of AB.
line. Hence, the line segment AB is symmetrical about its perpendicular bisector POQ.
16. Using a ruler and a compass, draw a line segment CD = 6 cm. With centre C and radius 3 cm, draw a
circle. With centre D and radius 3.5 cm, draw another circle. Where the two circles intersect, mark the points as P
P and Q.
17. Using your protractor, draw an angle of 112°. Bisect this angle using only a compass.
18. Using a ruler and a compass, construct a square of side 4 cm. A B
19. Using a rule and compass, construct a rectangle whose sides are 5 cm and 3 cm
20. Using a ruler and compass, construct the following angles.
a. 120° b. 60° C. 45° Q

EXAMPLE:2 A given angle having equal arms is symmetrical about the B
bisector of the angle.
Method Let AOB be a given angle with equal arms OA and OB, and
let OC be the bisector of AOB. C
Then, clearly AOC and BOC are identical.
Hence, AOB is symmetrical about the bisector OC.
O A
EXAMPLE:3 An isosceles triangle is symmetrical about the bisector of the
angle included between the equal sides.
Let ABC be an isosceles triangle in which AB = AC and let AD be
the bisector of BAC.
If ABC be folded along AD then ADC coincides exactly with
ADB.
Thus, ADC is identical with ADB.
Hence, AD is the line of symmetry of ABC.

EXAMPLE:4 A kite has one line of symmetry, namely, the diagonal shown
dotted in the adjotning figure.
Method Here ABCD is a kite in which AB = AD and BC = DC.
If we fold the kite along the line AC, we find that the two parts
coincide with each other
Hence, the kite ABCD is symmetrical about the diagonal AC.