Page 154 - classs 6 a_Neat

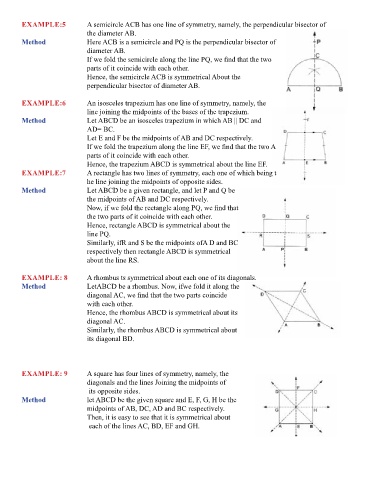
EXAMPLE:5 A semicircle ACB has one line of symmetry, namely, the perpendicular bisector of EXAMPLE:10 An equilateral triangle ts symmetrical about each one of the bisectors of its interior
the diameter AB. angles.
Method Here ACB is a semicircle and PQ is the perpendicular bisector of Method Let ABC be an equilateral triangle and let AD, BE and CF be the bisectors
diameter AB. of A, B
If we fold the semicircle along the line PQ, we find that the two and C respectively.
parts of it coincide with each other. Then, it is easy to see that ABC is symmetrical about each of the lines AD, BE and
Hence, the semicircle ACB is symmetrical About the CF.
perpendicular bisector of diameter AB.

EXAMPLE:6 An isosceles trapezium has one line of symmetry, namely, the
line joining the midpoints of the bases of the trapezium.
Method Let ABCD be an isosceles trapezium in which AB || DC and
AD= BC.
Let E and F be the midpoints of AB and DC respectively.
If we fold the trapezium along the line EF, we find that the two A
parts of it coincide with each other.
Hence, the trapezium ABCD is symmetrical about the line EF. EXAMPLE:11 A circle ts symmetrical about each of its diameters. Thus, each diameter of a
EXAMPLE:7 A rectangle has two lines of symmetry, each one of which being t circle ts an axis of symmetry.
he line joining the midpoints of opposite sides. Method Here, a number of diameters of a circle have been drawn. It is easy to see that the
Method Let ABCD be a given rectangle, and let P and Q be circle is symmetrical about each of the diameters drawn. Hence, a circle has an
the midpoints of AB and DC respectively. infinite number of lines of symmetry .
Now, if we fold the rectangle along PQ, we find that
the two parts of it coincide with each other.
Hence, rectangle ABCD is symmetrical about the
line PQ.
Similarly, ifR and S be the midpoints ofA D and BC
respectively then rectangle ABCD is symmetrical
about the line RS.

EXAMPLE: 8 A rhombus ts symmetrical about each one of its diagonals. REMARKS (i)A scalene triangle has no line of symmetry.
Method LetABCD be a rhombus. Now, ifwe fold it along the (ii)A parallelogram has no line of symmetry.
diagonal AC, we find that the two parts coincide
with each other. EXAMPLE:12 Each of the following capital letters of the English alphabet is symmetrical about
Hence, the rhombus ABCD is symmetrical about its the dotted line or lines as shown .
diagonal AC.
Similarly, the rhombus ABCD is symmetrical about
its diagonal BD. A B C D E

H I M O T
EXAMPLE: 9 A square has four lines of symmetry, namely, the
diagonals and the lines Joining the midpoints of
its opposite sides.
Method let ABCD be the given square and E, F, G, H be the U V W X Y
midpoints of AB, DC, AD and BC respectively.
Then, it is easy to see that it is symmetrical about
each of the lines AC, BD, EF and GH.
A B D O