Page 189 - classs 6 a_Neat

AREA SOLVED EXAMPLES

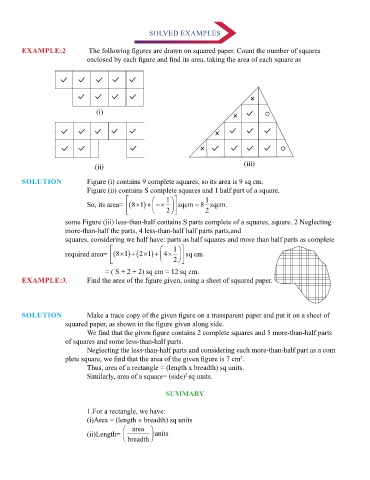
AREA The measurement of the region enclosed by a plane figure is called the area of EXAMPLE:2 The following figures are drawn on squared paper. Count the number of squares
the figure. enclosed by each figure and find its area, taking the area of each square as
STANDARD UNIT OF AREA We say that the area of a square of side 1 cm is 1cm
1 square centimetre, written as l cm . The standard unit of area is cm 2. 1cm 1cm
2
NOTE if a figure contains n squares, each of side 1 cm, we say that the 1cm

area of the figure is n cm .
2
EXAMPLE:1 Consider the following regions: (i)
S R

D D

(ii) (iii)

SOLUTION Figure (i) contains 9 complete squares, so its area is 9 sq cm.
Figure (ii) contains S complete squares and 1 half part of a square.
A B P Q  )  1   1
×
=


(i) (ii) So, its area= (8 1 + −×   sqcm 8 sqcm.
  2   2
In figure (1) ABCD is a rectangle containing 5 × 3 = 15 squares, each having an area of 1 cm . some Figure (iii) less-than-half contains S parts complete of a squares, square. 2 Neglecting
2
∴ area of rectangle ABCD = 15 × 1 cm2 = 15 cm . more-than-half the parts, 4 less-than-half half parts parts,and
2
In figure (ii) PQRS is a square containing 4 × 4 = 16 squares, each having an area of 1 cm squares, considering we half have: parts as half squares and more than half parts as complete

∴area of square PQRS = 16 × 1 cm = 16 cm. required area= (81×+ )  4× 1   sq cm
) (2 1×+
TO FIND AREA USING SQUARED PAPER     2    
Suppose we have to find the area of a given figure. = ( S + 2 + 2) sq cm = 12 sq cm.
We squared take a paper. trace copy of the figure on a transparent paper and place it on a sheet of EXAMPLE:3. Find the area of the figure given, using a sheet of squared paper.
Let the given figure enclose m complete squares, n more-than-half squares and p exactly
half squares.

Then, area of the figure = m n+ + 1  p cm 2


 2  SOLUTION Make a trace copy of the given figure on a transparent paper and put it on a sheet of
NOTE We half consider square. a more-than-half square as a complete square and neglect each less-than squared paper, as shown in the figure given along side.
half square. We find that the given figure contains 2 complete squares and 5 more-than-half parts
of squares and some less-than-half parts.
Neglecting the less-than-half parts and considering each more-than-half part as a com
plete square, we find that the area of the given figure is 7 cm .
2
Thus, area of a rectangle = (length x breadth) sq units.
Similarly, area of a square= (side) sq units.
2

SUMMARY

1.For a rectangle, we have:
(i)Area = (length × breadth) sq units
 area 
(ii)Length=   units
 breadth 