Page 191 - classs 6 a_Neat

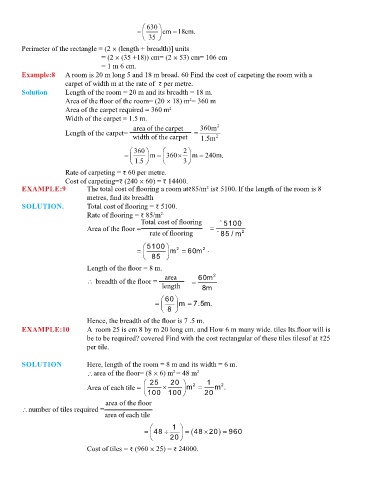
 630 
=
 area  =   cm 18cm.
(iii)Breadth=   units  35 
 length  Perimeter of the rectangle = (2 × (length + breadth)] units
(iv)Perimeter = 2 × (length + breadth) units = (2 × (35 +18)) cm= (2 × 53) cm= 106 cm
II. For a square, we have: = 1 m 6 cm.
 1 2  Example:8 A room is 20 m long 5 and 18 m broad. 60 Find the cost of carpeting the room with a
(i)Area = (side) sq units=  2 × (diagonal ) 
 2  carpet of width m at the rate of ₹ per metre.

Perimeter = ( 4 × side) units Solution Length of the room = 20 m and its breadth = 18 m.
2
In calculating the area of a rectangle, we must express the length and breadth in the Area of the floor of the room= (20 × 18) m = 360 m
Area of the carpet required = 360 m
2
SOLVED EXAMPLES Width of the carpet = 1.5 m. 2
Length of the carpet= area of the carpet = 360m
EXAMPLE:4 Find the area of a rectangle whose length and breadth are 45 cm and 18 cm width of the carpet 1.5m 2
respectively. Also.find the perimeter of the rectangle.  360   2 
SOLUTION Here, length= 45 cm and breadth= 18 cm. =  1.5   m =  360 × 3   m = 240m.
Area of the rectangle = (length x breadth) sq units  
= (45 × 18) cm= 810 cm . Rate of carpeting = ₹ 60 per metre.
2
Perimeter of the rectangle = (2 × (length + breadth)) units Cost of carpeting=₹ (240 × 60) = ₹ 14400.
2
same unit. EXAMPLE:9 The total cost of flooring a room at₹85/m is₹ 5100. lf the length of the room is 8
EXAMPLE:5 Find the area and perimeter of a rectangular plot of land whose length and metres, find its breadth
breadth are 28.5 m and 20 m respectively. SOLUTION. Total cost of flooring = ₹ 5100.
SOLUTION Here, length= 28.5 m and breadth= 20 m. Rate of flooring = ₹ 85/m 2.
∴ area of the plot = (length x breadth) sq units Area of the floor = Total cost of flooring = ` 5100

= (28.5 20 m× ) 2 =   285 × 20 m =   2 570m 2 rate of flooring ` 85 / m 2
 10    5100 2 2
Perimeter of the plot = [2 x (length + breadth] units =     85 m = 60m ⋅
= [2 X (28.5 + 20)] m Length of the floor = 8 m.

= (2 48.5 m× ) =   2 × 485   m 97m area 60m 2
=
 10  ∴ breadth of the floor = length = 8m
EXAMPLE:6 Find the cost of cultivating a rectangular field 80 m long and 35 m wide at ₹5 per
square metre. Also.find the cost of fencing the field at ₹24 per metre. =    60  m = 7.5m.
SOLUTION Length = 80 m and breadth = 35 m.   8
Area of the field = (length x breadth) sq units Hence, the breadth of the floor is 7 .5 m.
= ( 80 × 35)m = 2800 m 2 EXAMPLE:10 A room 25 is cm 8 by m 20 long cm. and How 6 m many wide. tiles Its.floor will is
2
Rate of cultivation =₹15 per m 2 be to be required? covered Find with the cost rectangular of these tiles tilesof at ₹25
∴ cost of cultivation = ₹(2800 × 15) = ₹ 42000. per tile.
Perimeter of the field = [2 × (length + breadth)) units
= (2 × (80 + 35)) m = (2 × 115) m= 230 m. SOLUTION Here, length of the room = 8 m and its width = 6 m.
Rate of fencing = ₹24 per metre. ∴area of the floor= (8 × 6) m = 48 m 2
2
∴ cost of fencing = ₹ (230 × 24) = ₹520.  20  25 1
2
EXAMPLE:7 The area of a rectangle is 630 cm Area of each tile =   × 100   100 m 2 = 20 m.
the rectangle.
SOLUTION Area = 630 cm and length = 35 cm. ∴number of tiles required = area of the floor
2
area of each tile
area in cm 2 60cm 2 1
Breadth of the rectangle = = =  ÷ 48  = (48 20 ) = 960
×
length in cm 35cm  
 20 
Cost of tiles = ₹ (960 × 25) = ₹ 24000.