Page 14 - Mechanics of Structures – Chapter 1

Mechanics of Civil Engineering Structures – Chapter 5

Solution

. .
Shear Stress Equation, τ =
.

Where,
20 (7)
1. S = Shear force = = = 70000 N
2 2
2. To determine from base and Ixx = ∑ = (200 10)(5) +(240 10)(130) = 73.18 mm from
⃐
∑ (200 10)+(240 10)
base

3. To determine Ixx = Second moment of area = Ixx1 + Ixx2
= ( 3 + A1d1 ) + ( 3 + A2d2 )
2
2
12 12
200 (10) 3 2 10 (240) 3
= ( 12 + (2000)(73.18-5) ) + ( 12 +
2
(2400)(130-73.18) )
= (9.32 x 10 ) + (19.26 x 10 ) mm 4
6
6
4
6
= 28.58 x 10 mm

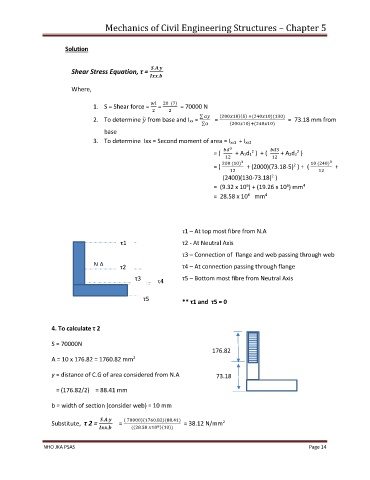
τ1 – At top most fibre from N.A

τ1 τ2 - At Neutral Axis
τ3 – Connection of flange and web passing through web

N.A
τ2 τ4 – At connection passing through flange
τ3 τ4 τ5 – Bottom most fibre from Neutral Axis

τ5
** τ1 and τ5 = 0

4. To calculate τ 2

S = 70000N
176.82
2
A = 10 x 176.82 = 1760.82 mm

y = distance of C.G of area considered from N.A 73.18

= (176.82/2) = 88.41 mm

b = width of section (consider web) = 10 mm

2
Substitute, τ 2 = . . = ( 70000)(1760.82)(88.41) = 38.12 N/mm
6
. ((28.58 10 )(10))

NHO JKA PSAS Page 14