Page 20 - swp0000.dvi

Morikawa [11] by introducing the scales



 =  ( − )   =   (1.9)

where ,  are the scaling parameters and  is a very small parameter.

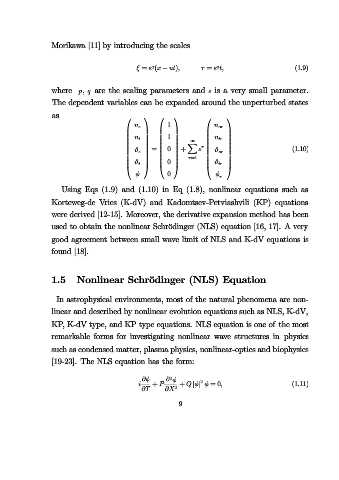
The dependent variables can be expanded around the unperturbed states
as ⎛ ⎞ ⎛ ⎞ ⎛ ⎞

1
   
⎜ ⎟ ⎜ ⎟ ⎜ ⎟
⎜ ⎟ ⎜ ⎟ ⎜ ⎟
⎜   ⎟ ⎜ 1 ⎟ ⎜   ⎟
⎜ ⎟ ⎜ ⎟ ∞ ⎜ ⎟
X
⎜ ⎟ = ⎜ 0 ⎟ +  ⎜ ⎟  (1.10)
⎟
⎟
⎜
⎟
⎜    ⎜  
⎜ ⎟ ⎜ ⎟ ⎜ ⎟
=1
⎜ ⎟ ⎜ ⎟ ⎜ ⎟
⎜   ⎟ ⎜ 0 ⎟ ⎜   ⎟
⎝ ⎠ ⎝ ⎠ ⎝ ⎠
 0 

Using Eqs (1.9) and (1.10) in Eq (1.8), nonlinear equations such as
Korteweg-de Vries (K-dV) and Kadomtsev-Petviashvili (KP) equations
were derived [12-15]. Moreover, the derivative expansion method has been
used to obtain the nonlinear Schrödinger (NLS) equation [16, 17]. A very
good agreement between small wave limit of NLS and K-dV equations is
found [18].

1.5 Nonlinear Schrödinger (NLS) Equation

In astrophysical environments, most of the natural phenomena are non-

linear and described by nonlinear evolution equations such as NLS, K-dV,
KP, K-dV type, and KP type equations. NLS equation is one of the most

remarkable forms for investigating nonlinear wave structures in physics

such as condensed matter, plasma physics, nonlinear-optics and biophysics
[19-23]. The NLS equation has the form:

2
  
2
 +  +  ||  =0 (1.11)
  2
9