Page 123 - PowerPoint 演示文稿

Bifocal Lenses 113

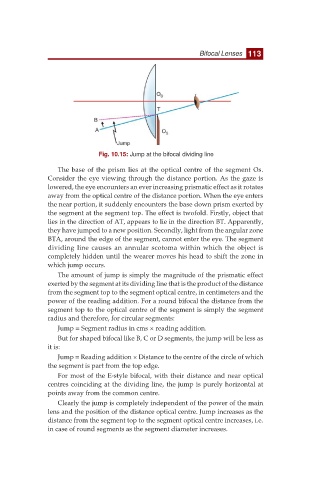
Fig. 10.15: Jump at the bifocal dividing line

The base of the prism lies at the optical centre of the segment Os.
Consider the eye viewing through the distance portion. As the gaze is
lowered, the eye encounters an ever increasing prismatic effect as it rotates
away from the optical centre of the distance portion. When the eye enters
the near portion, it suddenly encounters the base down prism exerted by
the segment at the segment top. The effect is twofold. Firstly, object that
lies in the direction of AT, appears to lie in the direction BT. Apparently,
they have jumped to a new position. Secondly, light from the angular zone
BTA, around the edge of the segment, cannot enter the eye. The segment
dividing line causes an annular scotoma within which the object is
completely hidden until the wearer moves his head to shift the zone in
which jump occurs.
The amount of jump is simply the magnitude of the prismatic effect
exerted by the segment at its dividing line that is the product of the distance
from the segment top to the segment optical centre, in centimeters and the
power of the reading addition. For a round bifocal the distance from the
segment top to the optical centre of the segment is simply the segment
radius and therefore, for circular segments:
Jump = Segment radius in cms × reading addition.
But for shaped bifocal like B, C or D segments, the jump will be less as
it is:
Jump = Reading addition × Distance to the centre of the circle of which
the segment is part from the top edge.
For most of the E-style bifocal, with their distance and near optical
centres coinciding at the dividing line, the jump is purely horizontal at
points away from the common centre.
Clearly the jump is completely independent of the power of the main
lens and the position of the distance optical centre. Jump increases as the
distance from the segment top to the segment optical centre increases, i.e.
in case of round segments as the segment diameter increases.