Page 40 - PowerPoint 演示文稿

30 Ophthalmic Lenses

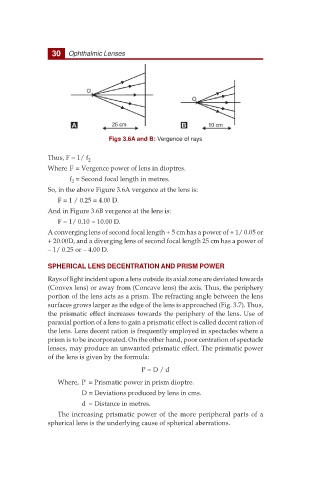
Figs 3.6A and B: Vergence of rays

Thus, F = 1/ f 2
Where F = Vergence power of lens in dioptres.
f = Second focal length in metres.
2
So, in the above Figure 3.6A vergence at the lens is:
F = 1 / 0.25 = 4.00 D.
And in Figure 3.6B vergence at the lens is:
F = 1/ 0.10 = 10.00 D.
A converging lens of second focal length + 5 cm has a power of + 1/ 0.05 or
+ 20.00D, and a diverging lens of second focal length 25 cm has a power of
– 1/ 0.25 or – 4.00 D.

SPHERICAL LENS DECENTRATION AND PRISM POWER

Rays of light incident upon a lens outside its axial zone are deviated towards
(Convex lens) or away from (Concave lens) the axis. Thus, the periphery
portion of the lens acts as a prism. The refracting angle between the lens
surfaces grows larger as the edge of the lens is approached (Fig. 3.7). Thus,
the prismatic effect increases towards the periphery of the lens. Use of
paraxial portion of a lens to gain a prismatic effect is called decent ration of
the lens. Lens decent ration is frequently employed in spectacles where a
prism is to be incorporated. On the other hand, poor centration of spectacle
lenses, may produce an unwanted prismatic effect. The prismatic power
of the lens is given by the formula:
P = D / d

Where, P = Prismatic power in prism dioptre.
D = Deviations produced by lens in cms.
d = Distance in metres.
The increasing prismatic power of the more peripheral parts of a
spherical lens is the underlying cause of spherical aberrations.