Page 125 - Mathematics Coursebook

12.4 Constructing squares, rectangles and polygons

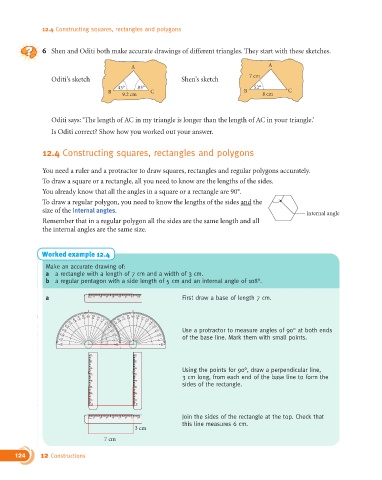
6 Shen and Oditi both make accurate drawings of different triangles. They start with these sketches.

A A A A

7 cm
Oditi’s sketch Shen’s sketch 7 cm
43° 83° 43° 52° 83° 52°
B C B B C C B C
9.2 cm 9.2 cm 8 cm 8 cm

Oditi says: ‘The length of AC in my triangle is longer than the length of AC in your triangle.’

Is Oditi correct? Show how you worked out your answer.

12.4 Constructing squares, rectangles and polygons

You need a ruler and a protractor to draw squares, rectangles and regular polygons accurately.

To draw a square or a rectangle, all you need to know are the lengths of the sides.
You already know that all the angles in a square or a rectangle are 90°.
To draw a regular polygon, you need to know the lengths of the sides and the
size of the internal angles. internal angle
Remember that in a regular polygon all the sides are the same length and all
the internal angles are the same size.

Worked example 12.4

Make an accurate drawing of:
a a rectangle with a length of 7 cm and a width of 3 cm.
b a regular pentagon with a side length of 5 cm and an internal angle of 108°.

a 1 2 3 4 5 6 7 8 First draw a base of length 7 cm.

70 100 80 70 80 100
60 110 90 80 70 60 60 110 90 80 70 60
50 120 100 110 120 130 140 50 120 100 110 120 130 140
40 140 130 50 40 40 40 40 140 140 130 50 40
150 140
30 150 3 30 30 30 30 30 150 Use a protractor to measure angles of 90° at both ends
20 160 20 2 20 160 15 5 160 150 150 20 160 20 160 of the base line. Mark them with small points.
10 170 10 10 0 0 170 160 170 10 170 10 170
0 180 0 0 180 17 180 0 180 0 180
8 8
7 7
Using the points for 90°, draw a perpendicular line,
6 6
3 cm long, from each end of the base line to form the
5 5
4 4
sides of the rectangle.
3 3
2 2
1 1
Join the sides of the rectangle at the top. Check that
1 2 3 4 5 6 7 8
this line measures 6 cm.
3 cm
7 cm

124 12 Constructions