Page 160 - Mathematics Coursebook

16.4 Estimating probabilities

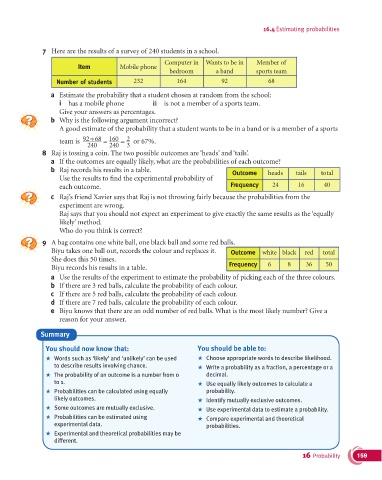
7 Here are the results of a survey of 240 students in a school.
Computer in Wants to be in Member of
Item Mobile phone
bedroom a band sports team
Number of students 232 164 92 68

a Estimate the probability that a student chosen at random from the school:
i has a mobile phone ii is not a member of a sports team.
Give your answers as percentages.
b Why is the following argument incorrect?
A good estimate of the probability that a student wants to be in a band or is a member of a sports
+
2
team is 92 68 = 160 = or 67%.
240 240 3
8 Raj is tossing a coin. The two possible outcomes are ‘heads’ and ‘tails’.
a If the outcomes are equally likely, what are the probabilities of each outcome?
b Raj records his results in a table. Outcome heads tails total
Use the results to find the experimental probability of

each outcome. Frequency 24 16 40
c Raj’s friend Xavier says that Raj is not throwing fairly because the probabilities from the
experiment are wrong.
Raj says that you should not expect an experiment to give exactly the same results as the ‘equally
likely’ method.
Who do you think is correct?

9 A bag contains one white ball, one black ball and some red balls.
Biyu takes one ball out, records the colour and replaces it. Outcome white black red total
She does this 50 times.
Biyu records his results in a table. Frequency 6 8 36 50
a Use the results of the experiment to estimate the probability of picking each of the three colours.
b If there are 3 red balls, calculate the probability of each colour.
c If there are 5 red balls, calculate the probability of each colour.
d If there are 7 red balls, calculate the probability of each colour.
e Biyu knows that there are an odd number of red balls. What is the most likely number? Give a
reason for your answer.

Summary

You should now know that: You should be able to:
+ Words such as ‘likely’ and ‘unlikely’ can be used + Choose appropriate words to describe likelihood.
to describe results involving chance. + Write a probability as a fraction, a percentage or a
+ The probability of an outcome is a number from 0 decimal.
to 1. + Use equally likely outcomes to calculate a
+ Probabilities can be calculated using equally probability.
likely outcomes. + Identify mutually exclusive outcomes.
+ Some outcomes are mutually exclusive. + Use experimental data to estimate a probability.
+ Probabilities can be estimated using + Compare experimental and theoretical
experimental data. probabilities.
+ Experimental and theoretical probabilities may be
different.

16 Probability 159