Page 204 - Servo Motors and Industrial Control Theory

Appendix B 201

28. This problem is a new idea. Assume that in problem 27 a steady state force is
available to give the train a steady speed. In addition assume that some varia-
tion of force can be implemented to control the vibration of the wagons. Sup-
pose that the displacement and velocity of each wagon is measurable. Remove
the dampers from the system and design a state variable feedback control strat-
egy so that all eigenvalues are moved to the required positions on the s-plane.
You choose where the eigenvalues should be located on the s-plane. They must
not be too fast because the variation of the applied force might not be so fast.
Remember that before implementing state variable feedback control strategy
you must check that the system is controllable with respect to the variation of
the particular input.
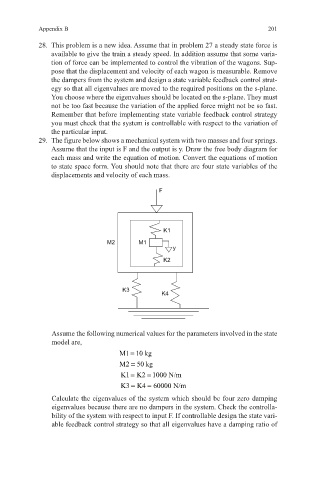
29. The figure below shows a mechanical system with two masses and four springs.
Assume that the input is F and the output is y. Draw the free body diagram for
each mass and write the equation of motion. Convert the equations of motion
to state space form. You should note that there are four state variables of the
displacements and velocity of each mass.
F

K1
M2 M1
y
K2

K3
K4

Assume the following numerical values for the parameters involved in the state
model are,
M1 10 kg=
M2 = 50 kg
K1 K2 1000 N/m= =
K3 = K4 = 60000 N/m
Calculate the eigenvalues of the system which should be four zero damping
eigenvalues because there are no dampers in the system. Check the controlla-
bility of the system with respect to input F. If controllable design the state vari-
able feedback control strategy so that all eigenvalues have a damping ratio of