Page 151 - Fiber Optic Communications Fund

132 Fiber Optic Communications

(c) The mean photon density and the current are related by Eq. (3.133),
(I − I ) ph
th
N =
ph
qV
or
N qV
ph
I = I +
th
ph
21
8.5 × 10 × 1.602 × 10 −19 × 4.5 × 10 −16
−3
= 52.7 × 10 +
2.13 × 10 −12
= 340.4 mA.

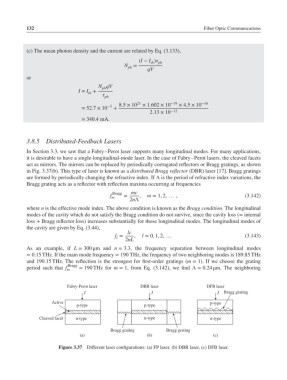
3.8.5 Distributed-Feedback Lasers
In Section 3.3, we saw that a Fabry–Perot laser supports many longitudinal modes. For many applications,
it is desirable to have a single-longitudinal-mode laser. In the case of Fabry–Perot lasers, the cleaved facets
act as mirrors. The mirrors can be replaced by periodically corrugated reflectors or Bragg gratings, as shown
in Fig. 3.37(b). This type of laser is known as a distributed Bragg reflector (DBR) laser [17]. Bragg gratings
are formed by periodically changing the refractive index. If Λ is the period of refractive index variations, the
Bragg grating acts as a reflector with reflection maxima occurring at frequencies
Bragg mc
f m = , m = 1, 2, … , (3.142)
2nΛ
where n is the effective mode index. The above condition is known as the Bragg condition. The longitudinal
modes of the cavity which do not satisfy the Bragg condition do not survive, since the cavity loss (= internal
loss + Bragg reflector loss) increases substantially for those longitudinal modes. The longitudinal modes of
the cavity are given by Eq. (3.44),
lc
f = , l = 0, 1, 2, … (3.143)
l
2nL
As an example, if L = 300 μm and n = 3.3, the frequency separation between longitudinal modes
= 0.15 THz. If the main mode frequency = 190 THz, the frequency of two neighboring modes is 189.85 THz
and 190.15 THz. The reflection is the strongest for first-order gratings (m = 1). If we choose the grating
Bragg
period such that f = 190 THz for m = 1, from Eq. (3.142), we find Λ= 0.24 μm. The neighboring
m

Fabry-Perot laser DBR laser DFB laser
I I I Bragg grating
Active p-type
p-type p-type

Cleaved facet n-type n-type n-type
Bragg grating Bragg grating
(a) (b) (c)

Figure 3.37 Different laser configurations: (a) FP laser, (b) DBR laser, (c) DFB laser.