Page 49 - Fiber Optic Communications Fund

30 Fiber Optic Communications

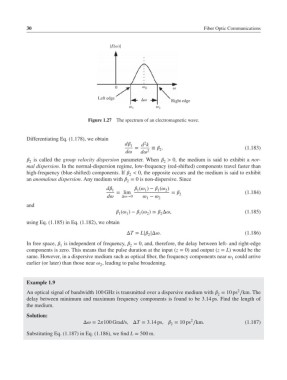
∣E(ω)∣

0 ω 0 ω
Left edge Δω Right edge
ω 1 ω 2

Figure 1.27 The spectrum of an electromagnetic wave.

Differentiating Eq. (1.178), we obtain
d 1 d k
2
= ≡ . (1.183)
2
d d 2
is called the group velocity dispersion parameter. When > 0, the medium is said to exhibit a nor-
2
2
mal dispersion. In the normal-dispersion regime, low-frequency (red-shifted) components travel faster than
high-frequency (blue-shifted) components. If < 0, the opposite occurs and the medium is said to exhibit
2
an anomalous dispersion. Any medium with = 0 is non-dispersive. Since
2
d 1 ( )− ( )
2
1
1
1
= lim = 2 (1.184)
d Δ→0 − 2
1
and
( )− ( )≃ Δ, (1.185)
1 1 1 2 2
using Eq. (1.185) in Eq. (1.182), we obtain
ΔT = L| |Δ. (1.186)
2
In free space, is independent of frequency, = 0, and, therefore, the delay between left- and right-edge
2
1
components is zero. This means that the pulse duration at the input (z = 0) and output (z = L) would be the
same. However, in a dispersive medium such as optical fiber, the frequency components near could arrive
1
earlier (or later) than those near , leading to pulse broadening.
2
Example 1.9
2
An optical signal of bandwidth 100 GHz is transmitted over a dispersive medium with = 10 ps ∕km. The
2
delay between minimum and maximum frequency components is found to be 3.14 ps. Find the length of
the medium.
Solution:
2
Δ = 2100 Grad/s, ΔT = 3.14 ps, = 10 ps ∕km. (1.187)
2
Substituting Eq. (1.187) in Eq. (1.186), we find L = 500 m.