Page 50 - FINAL CFA I SLIDES JUNE 2019 DAY 3
P. 50
Session Unit 3:
LOS 10.l: Define the standard normal distribution,
explain how to standardize a RV, and calculate and 10. Common Probability Distributions
interpret probabilities using the standard normal
distribution, p.226
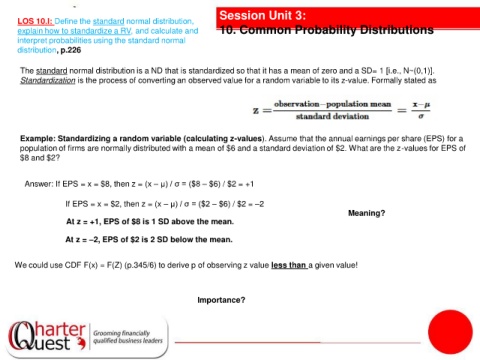
The standard normal distribution is a ND that is standardized so that it has a mean of zero and a SD= 1 [i.e., N~(0,1)].
Standardization is the process of converting an observed value for a random variable to its z-value. Formally stated as
Example: Standardizing a random variable (calculating z-values). Assume that the annual earnings per share (EPS) for a
population of firms are normally distributed with a mean of $6 and a standard deviation of $2. What are the z-values for EPS of
$8 and $2?
Answer: If EPS = x = $8, then z = (x – μ) / σ = ($8 – $6) / $2 = +1
If EPS = x = $2, then z = (x – μ) / σ = ($2 – $6) / $2 = –2
Meaning?
At z = +1, EPS of $8 is 1 SD above the mean.
At z = –2, EPS of $2 is 2 SD below the mean.
We could use CDF F(x) = F(Z) (p.345/6) to derive p of observing z value less than a given value!
Importance?