Page 30 - PowerPoint Presentation

READING 8: MULTIPLE REGRESSION AND ISSUES IN REGRESSION ANALYSIS
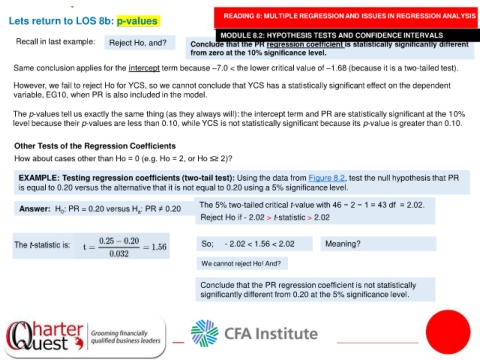
Lets return to LOS 8b: p-values

MODULE 8.2: HYPOTHESIS TESTS AND CONFIDENCE INTERVALS
Recall in last example: Reject Ho, and? Conclude that the PR regression coefficient is statistically significantly different
from zero at the 10% significance level.

Same conclusion applies for the intercept term because –7.0 < the lower critical value of –1.68 (because it is a two-tailed test).

However, we fail to reject Ho for YCS, so we cannot conclude that YCS has a statistically significant effect on the dependent
variable, EG10, when PR is also included in the model.

The p-values tell us exactly the same thing (as they always will): the intercept term and PR are statistically significant at the 10%
level because their p-values are less than 0.10, while YCS is not statistically significant because its p-value is greater than 0.10.

Other Tests of the Regression Coefficients

How about cases other than Ho = 0 (e.g. Ho = 2, or Ho ≤≥ 2)?

EXAMPLE: Testing regression coefficients (two-tail test): Using the data from Figure 8.2, test the null hypothesis that PR
is equal to 0.20 versus the alternative that it is not equal to 0.20 using a 5% significance level.

Answer: H : PR = 0.20 versus H : PR ≠ 0.20 The 5% two-tailed critical t-value with 46 − 2 − 1 = 43 df = 2.02.
a
0
Reject Ho if - 2.02 > t-statistic > 2.02

So; - 2.02 < 1.56 < 2.02 Meaning?

We cannot reject Ho! And?

Conclude that the PR regression coefficient is not statistically
significantly different from 0.20 at the 5% significance level.