Page 247 - J. C. Turner "History and Science of Knots"

238 History and Science of Knots

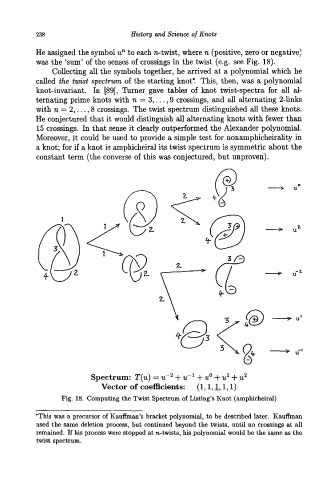
He assigned the symbol u" to each n-twist, where n (positive, zero or negative)
was the `sum' of the senses of crossings in the twist (e.g. see Fig. 18).
Collecting all the symbols together, he arrived at a polynomial which he
called the twist spectrum of the starting knot*. This, then, was a polynomial
knot-invariant. In [89], Turner gave tables of knot twist-spectra for all al-
ternating prime knots with n = 3, ... , 9 crossings, and all alternating 2-links
with n = 2,.. . , 8 crossings. The twist spectrum distinguished all these knots.
He conjectured that it would distinguish all alternating knots with fewer than
15 crossings. In that sense it clearly outperformed the Alexander polynomial.
Moreover, it could be used to provide a simple test for nonamphicheirality in
a knot; for if a knot is amphicheiral its twist spectrum is symmetric about the
constant term (the converse of this was conjectured, but unproven).

U 0

2.
u

- ; u Z

Spectrum: T(u) = u-2 + u-1 + u° + u1 + u2
Vector of coefficients: (1,1,1,1,1)
Fig. 18. Computing the Twist Spectrum of Listing's Knot (amphicheiral)

*This was a precursor of Kauffman's bracket polynomial , to be described later. Kauffman
used the same deletion process , but continued beyond the twists, until no crossings at all
remained. If his process were stopped at n-twists, his polynomial would be the same as the
twist spectrum.