Page 249 - J. C. Turner "History and Science of Knots"

240 History and Science of Knots

parallel strings in a plane, all hanging vertically from a line drawn on, for
example, the ceiling, and dropping down to a line on the floor. There are thus
2n string endpoints, n on the ceiling and n on the floor. In a given braid,
the endpoints are all to be regarded as fixed. This first configuration, with all
strings parallel, is the null braid; it acts as the unit element of the geometric
braid group B(n). If now the lower endpoints are removed from the floor, the
strings interwoven in some manner, and finally the endpoints are refixed to the
floor in some order, then a new n-string braid will be achieved; such are the
members of B(n). With this geometric picture in mind, it is easy to imagine
the concatenation operation, which joins two braids `one on top of the other'.

1 2 3 2 3
1 2 3 4
01 Q2
-1
02

02 01

a2

a1 02

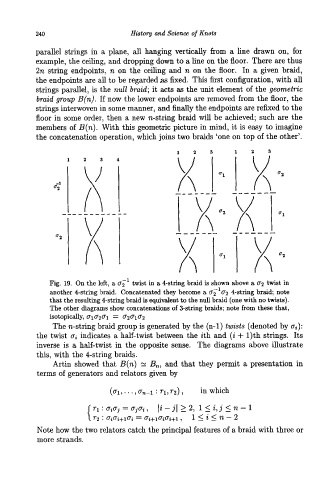
Fig. 19. On the left, a U2 1 twist in a 4-string braid is shown above a 0`2 twist in
another 4-string braid. Concatenated they become a o'2 1Q2 4-string braid; note
that the resulting 4-string braid is equivalent to the null braid (one with no twists).
The other diagrams show concatenations of 3-string braids; note from these that,
isotopically, 0`1o2o1 = a2u1o2
The n-string braid group is generated by the (n-1) twists (denoted by vi):
the twist of indicates a half-twist between the ith and (i + 1)th strings. Its
inverse is a half-twist in the opposite sense. The diagrams above illustrate
this, with the 4-string braids.
Artin showed that B(n) - Bn, and that they permit a presentation in
terms of generators and relators given by

(o1, • • • , an_1 : r1i r2), in which

^r1:uoj =aoi, Ji - jJ > 2, 1<i,j<n-1
r2 : a a2+1oz = 0`i+iai0ri+1 , 1 < i < n - 2
Note how the two relators catch the principal features of a braid with three or
more strands.