Page 246 - C:\Users\azizu\Documents\Flip PDF Professional\Matematics-Form-1-1\

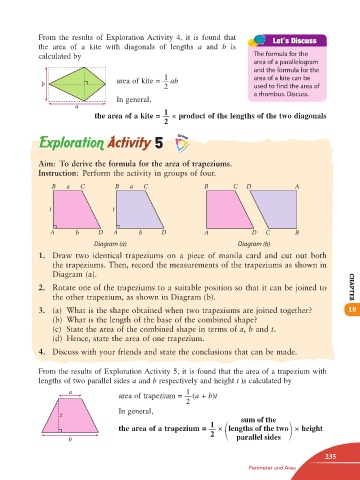
From the results of Exploration Activity 4, it is found that
the area of a kite with diagonals of lengths a and b is
calculated by The formula for the
area of a parallelogram
and the formula for the
1 area of a kite can be
b area of kite = ab
2 used to find the area of
a rhombus. Discuss.
In general,
a
1
the area of a kite = × product of the lengths of the two diagonals
2
5 Group

Aim: To derive the formula for the area of trapeziums.
Instruction: Perform the activity in groups of four.
B a C B a C B C D A

t t

A b D A b D A D C B
Diagram (a) Diagram (b)
1. Draw two identical trapeziums on a piece of manila card and cut out both
the trapeziums. Then, record the measurements of the trapeziums as shown in
Diagram (a).
2. Rotate one of the trapeziums to a suitable position so that it can be joined to CHAPTER
the other trapezium, as shown in Diagram (b).

3. (a) What is the shape obtained when two trapeziums are joined together? 10
(b) What is the length of the base of the combined shape?
(c) State the area of the combined shape in terms of a, b and t.
(d) Hence, state the area of one trapezium.
4. Discuss with your friends and state the conclusions that can be made.

From the results of Exploration Activity 5, it is found that the area of a trapezium with
lengths of two parallel sides a and b respectively and height t is calculated by
a 1
area of trapezium = (a + b)t
2
t In general, sum of the
2
2 1
1
the area of a trapezium = × lengths of the two × height
b parallel sides
235
Perimeter and Area

10 TB Math F1.indd 235 11/10/16 12:19 PM