Page 69 - MATHEMATICS COURSE FOR SECONDARY SCHOOLS BOOK 2

e
P n
e
a
2
a a o
e
u
If however his line of sight is declined at the correct
Or the angle of depression is the angle mea
sured downwards from the horizontal to an
Pm n
o
i
angle and he looks downwards then he would be able angle and he looks downwards then he would be able
,
sured downwards from the horizontal to an object.
object.
• Or, the angle of depression is the angle
• Or, the angle of depression is the angle between the line of sight of a person looking
between the line of sight of a person looking downwards and the horizontal.
to see Person A. The angle formed by Person B’s to see Person A. The angle formed by Person B’s
horizontal line of sight and his declined line of sight horizontal line of sight and his declined line of sight
is called the angle of depression. is called the angle of depression.
(c)
Horizontal plane
b
Horizontal plane
For example:
(c)
Horizontal plane
downwards and the horizontal.
b
Fig 11 80 Angles of elevation depression
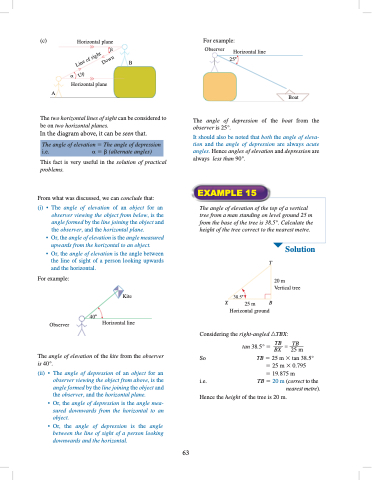
The two horizontal lines of sight can be considered to
For example:
B
Observer
Horizontal line
Observer Horizontal line
B
258 258
A Horizontal plane A
Boat Boat
The two horizontal lines of sight can be considered to be on two horizontal planes.
be on two horizontal planes.
From Fig. 11.80 above, it can be seen that.
)
This fact is very useful in the solution of practical
This fact is very useful in the solution of practical problems.
problems.
From what was discussed, we can conclude that: Chapter11.indd 593
Fig. 11.82 Angle of depression
Fig. 11.82 Angle of depression
Chapter11.indd 593
The angle of elevation of the top of a vertical
..
Fig. 11.80 Angles of elevation depression
IFnrotmheFdigia.g1r1a.8m0above,,itcanbbeeseseennthtahta.t.
The angle of elevation 5 The angle of depression
The angle of depression of the boat from the The angle of depression of the boat from the
observer is 25°. observer is 25°.
It should also be noted that both the angle of eleva- It should also be noted that both the angle of eleva-
The angle of elevation 5 The angle of depression
i.e.
i.e. a 5 b (alternate angles a 5 b (alternate angles)
(i) • The angle of elevation of an object for an
12/9/2009
5 46 m. The angle of
5 The an
observer viewing the object from below, is the angle formed by the line joining the object and the observer, and the horizontal plane.
Or, the angle of elevation is the angle measured upwards from the horizontal to an object.
12/9/2009 4:30:01 PM
tion and the angle of depression are always acute tion and the angle of depression are always acute
angles. Hence angles of elevation and depression are angles. Hence angles of elevation and depression are
always less than 90°. always less than 90°.
E
Mathematics: ACompleteCourse 593 Mathematics: ACompleteCourse 593
E
LE 15
The height of TB 5 (1.0 1
4:30:01 PM
5 55°. Considering t
t
So
Hence the dis building is 32
Example
A surveyor st on which an of elevation t 55° and 51° r the aerial.
Ae 142.8
T
X
xa
A
m
M
pl
P
e
of sight,
he correct ld be able erson A’s e of sight
• Or, the angle of elevation is the angle between the line of sight of a person looking upwards and the horizontal.
Solution
20 m Vertical tree
Fig. 11.83 Right-angled triangle
•
6
For example:
Observer
Kite
408
Horizontal line
38.58
X 25 m B
Horizontal ground
tree from a man standing on level ground 25 m from the base of the tree is 38.5°. Calculate the height of the tree correct to the nearest metre.
Fig. 11.81 Angle of elevation
The angle of elevation of the kite from the observer is 40°.
Considering the right-angled nTBX:
TB TB
(ii) • The angle of depression of an object for an observer viewing the object from above, is the of sight, angle formed by the line joining the object and
the observer, and the horizontal plane.
he correct • Or, the angle of depression is the angle mea-
ld be able sured downwards from the horizontal to an erson B’ s object.
e of sight • Or, the angle of depression is the angle between the line of sight of a person looking
downwards and the horizontal.
For example:
Horizontal line
So i.e.
tan 38.5° 5 } 5 } BX 25 m
TB 5 25 m 3 tan 38.5° 5 25 m 3 0.795
5 19.875 m
TB 5 20 m (correct to the nearest metre).
Observer
63
Hence the height of the tree is 20 m. Example
A girl 1.0 m in height, standing on top of a vertical building 45.0 m high sees a car some distance away when the angle of depression is 55°. What distance is the car from the base of the building?
258
T
Fig. 11.85 R
Girl 1.0 m
558
Line of sight
Line of sight
a
a
Up Up Down Down
T