Page 70 - MATHEMATICS COURSE FOR SECONDARY SCHOOLS BOOK 2

r n
l
}
r
o
o i
c
7
5
a h i
5 a
20 m
55° and 51° respectively Calculate the height of
2358m 1373m latethe
5 19.875 m
Vertical tree
1.428
i.e.
Hence the height of the tree is 20 m.
5 32.2 m (correct to 3 s.f.) T
TB 5 20 m (correct to the
38.58
X 25 m B
the aerial.
Hence the distance of the car from the base of the
builAdeinrigali1s93.32.m2m. A
nearest metre). Horizontal ground
E
EXAMPLE 17
Ex
PLE 16
X
a
A
m
Fig. 11.83 Right-angled triangle
22
M
ple
Example
Considering the right-angled nTBX:
142.8 m
123.5 m
A girl 1.0 m in height, standing on top oTfBa TB tan 38.5° 5 } 5 }
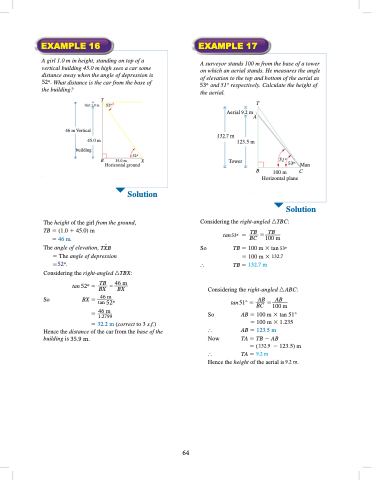
A surveyor stands 100 m from the base of a tower on which an aerial stands. He measures the angle
BX 25 m vertical building 45.0 m high sees a car some
Tower
distanceSaoway when the angle oTf Bde5pr2es5simon3istan 38.5° 552°o. What distance is the car from t5he2b5asme o3f 0.795
558 Man
of elevation to the top and bottom of the aerial as
the building?
i.e. T
535o° and 51° respectively. Calculate the height of
5 19.875 m
TB 5 20 m (correct to the Fig. 11.85 Right-angled triangle
the aerial.
Horizontal plane
BC
nearest metre).
T
Aerial 91.92.3mm6
A Solution
Considering the right-angled nTBC: 1432.87 m 123.5 m
So
[
5528o
Hence the height of the tree is 20 m.
Girl 1.0 m
46 m Vertical
Example
45.0 m
TB TB tan55°5 } 5}
A girl 1.0 m in height, standing on top of a
BC 100 m TB5T1o0w0emr 3tan55°
building
vertical building 45.0 m high s5e25oe8s a car some
B 35.0m X
distance away when the angle of depression is
53o
.
Fig. 11.84 Right-angled triangle
Horizontal ground
558 Man BC
55° What distance is the car from the base of
5 100 m 3 1.428
TB 5 142.8 m Horizontal plane
tical d 25 m
late the metre.
ution
ee
Bm
n 38.5°
.795
rect to the
est metre).
fa some
sion is
BC 100 m TB 5 100 m 3 tan 535o°
11/17/2009
11:36:43 PM
594
Trigonometry 1
Considering the right-angled nTBC:
So
[
the building?
The height of the girl from the ground,
558
B Horizontal ground X
45.0 m Chapter11.indd 594 building
TB 5 (1.0 1 45.0) m 5 46 m.
TB TB tan535o°5 } 5}
The angle of elevation, TXB
5 The angle of depression
5 100 m 3 13.422.78 TB 5 1432.78 m
Considering the right-angled n ABC:
5525o°..
Considering the right-angled nTBX:6
6T
Fig. 11.85 Right-angled triangle
6
Chapter 11
46 m Vertical
Girl 1.0
m 558 Solution
Solution
Fig. 11.84 Right-angled triangle
TB 46 m tan552°o5 } 5} BX BX
46 m
Solution
So
Example 22
A surveyor stands 100 m from the base of a tower on which an aerial stands. He measures the angle of elevation to the top and bottom of the aerial as 55° and 51° respectively. Calculate the height of the aerial.
T
Aerial 19.3 m
142.8 m
123.5 m
Tower
594
Chapter 11 Trigonometry 1
AB AB BC 100 m
1. A sail elevat cliff is 11:36:43 PMdistan metre.
2.
Fig. 11.8
The di situate AB 5 BAC
(a) th (b) th to
3. From from t elevat
BX 5 }
tan5528o tan51°5}5}
A
From a coastal lookout point P, 100 m above the sea, a sailor sights two boats A and B in the same direction. The angles of depression of the two boats are 15° and 23° respectively. Calculate the distance between the two boats.
46 m
So
[
Now
5}
AB 5 100 m 3 tan 51° 5 100 m 3 1.235
AB 5 123.5 m TA 5 TB 2 AB
Hence the height of the aerial is 91.92.m3 .m. Example 23
1.2472989
5 32.2 m (correct to 3 s.f.)
11/17/2009
Chapter11.indd 594
Hence the distance of the car from the base of the building is 325.29 m..
132.9
64
5 (142.8 2 123.5) m [ TA519.92.3mm
P
100 m
C
158
Horizontal plane
158
Horizontal plane A
558 Man
32.2 m
32.2 m
238
238
518
518
518
100 m
100 m
B