Page 71 - MATHEMATICS COURSE FOR SECONDARY SCHOOLS BOOK 2

Now TA 5 TB AB
11 A woman standing 20 m away from a tower
n
s e
s o
5 (142.8 2 123.5) m [ TA 5 19.3 m
2.
Hence the height of the aerial is 19.3 m. Considering the right-angled n ABC:
15 m 448
Vertical tower
EXERA CISE 3C.13 Exercise 11f
23
Example AB AB EXAMPLE 18
Fig. 11.87 Right-angled triangle
tan51°5} 5} BC 100 m
1. A sailor sights the top of a cliff at an angle of elevation of 152o°. He knows that the height of the
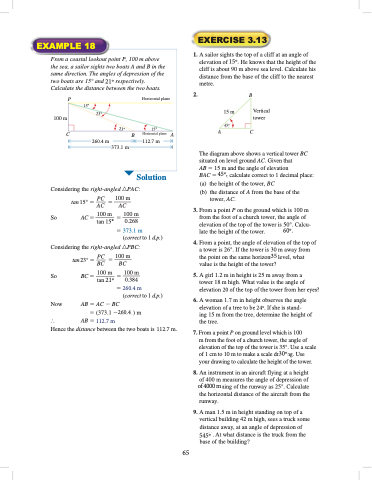
From a coastal lookout point P, 100 m above
The diagram above shows a vertical tower BC cliff is about 90 m above sea level. Calculate his
So AB 5 100 m 3 tan 51°
the sea, a sailor sights two boats A and B in the
samedirection.T5he1a0n0glmes3of1d.e2p3r5essionofthe [two boats areA1B5°5an1d232.315°omrespectively. Calculate the distance between the two boats.
P
Hence the height of the aerial is 19.3 m.
situated on level ground AC. Given that distance from the base of the cliff to the nearest
Now
TA 5 TB 2 AB
5 (142.8 2 123.5) mHorizontal plane
BAC 5 44°, calculate correct to 1 decimal place: 2. (a) the height of the tower, BC
AB 5 15 m and the angle of elevation metre.
[
100 m
Example 23 26305.48 m
15 m
tower
T15A8 519.3m
(b) the distance of A from the base of the tower, AC. Vertical
C
A
elevation of the top of the tower is 50°. Calcu- late the height of the tower.
11327..73m From a coastal lookout point P, 100 m above
Fig. 11.87 Right-angled triangle
158 Horizontal plane
448
from the foot of a church tower, the angle of
3. From a point P on the ground which is 100 m
4. From a point, the angle of elevation of the top of The diagram above shows a vertical tower BC
45o
AC
373.1 m
the sea, a sailor sights two boats A and B in the Fig. 11.86 Right-angled triangle
a tower is 26°. If the tower is 30 m away from
same direction. The angles of6
situated on level ground AC. Given that
the point on the same horizontal level, what
depression of the two boats are 15° and 23° respectively.
AB 5 15 m and the angle of elevation valueis4t5hoe,heightofthetower?
Calculate the distance between the tSwo lbuoattiso. n
BAC 5 44°, calculate correct to 1 decimal place: (a) the height of the tower, BC
ConsidPering the right-angled nPAC: Horizontal plane
5. A girl 1.2 m in height is 25 m away from a t(obw) etrhe18dimstahnicgeh.oWf Ahaftrovmalutheeisbathse aonf gthle of
100m
So
AC 5 }100 m tan 15􏰀
5 }100 m 0.268
158 Horizontal plane
36.FArowmomaapnoi1n.t7Pmoinntheigrhotuonbdsewrvheicshthise1a0n0glme forfoemletvhaetifonotoof faatrceheutrochbeto2w4e°.r,Ifthseheanigs lsetaonfd- einlegv1a5tiomn forfotmhethtoeptroefe,thdeteorwmeirniest5h0e°h. eCigahlctuo-f late the height of the tower. 60o.
So
5. A girl 1.2 m in height is 25 m away from a
8. tAown einrs1tr8umehnitgihn. Wanhaaitrcvralfutefliysinthgeaatnaghleiogfht
C
5 373.1 m137.3 m 373.1(mcorrect to 1 d.p.)
A
158
tan15°5} 5}
BC 5 } 5 } Considering the rigthatn-a2n3g􏰀led n0P.34A82C4:
PC 100 m AC AC
tower, AC.
elevation of the top of the tower from her eyes?
235.8 m
the tree.
47. From a poiintt,Pthoen agnrogulendoflevlelvwathioicnhoifs t1h0e0top of
Considering the right-angled nPBC: Fig. 11.86 Right-angled triangle
amtofrwomertihse2f6o°o.tIoffthaechtouwrcehrtiosw3e0r,mthaewaanyglferomf
PC 100 tan23°5} 5}
tehlevpaotiionnt ofntthheetosapmoef thoertiozwonet3ra5ilsl3ev5e°.l,Uwsehat scale
21o
6m
BC BC Solution
vofal1uecmisttohe10hemigthotmofaktheeatsocwaler?drawing. Use
100 m 100 m
your drawing to calculate the height of the tower.
tan15°5}PC5}15002m6305.48m
AC AC(correct to 1 d.p.)
eolfe4va0at0iomn 2om0fetoahfseuthtroepstotohpfetohafnetghtloewtoefwrdfeeropfmreoshmseiorhneyroefesy?es?
Now
AB 5 A10C02mBC 100 m AC 5 } 5 }
6. A woman 1.7 m in height observes the angle the horizontal distance of the aircraft from the oelfeevlaetvioantionf aoftraeetrteoe btoe 2b4eo2. 4If°s. hIfe sishestiasnsdt-and- runway.
So
tan 15􏰀 0.268 5 (373.1 2226305.4.8) m
the beginning of the runway as 25°. Calculate
Chapter11.indd
So
BC 5 }100 m tan 23􏰀
5 }100 m 0.424
5 235.8 m
(correct to 1 d.p.)
your drawing to calculate the height of the tower.
11/17/2009
8. An instrument in an aircraft flying at a height of 400 m measures the angle of depression of
9. A man 1.5 m in height standing on top of a
vertical building 42 m high, sees a truck some
distance away, at an angle of depression of
Mathematics: A Complete Course 595 base of the building?
11:36:44 PM
[
AB 5 11327.73 m5 373.1 m
(correct to 1 d.p.)
ing 15 m from the tree, determine the height of 9. tAhemtraene.1.5 m in height standing on top of a
Hence the distance between the two boats is 13172.37 m. Considering the right-angled nPBC:
vertical building 42 m high, sees a truck some
595
PC 100 m tan23°5} 5}
m from the foot of a church tower, the angle of
BC
BC
Mathematics: A Complete Course 595 of 1 cm to 10 m to make a scale dra3w0oing. Use
7. From a point P on ground level which is 100
elevation of the top of the tower is 35°. Use a scale
of 4000 m
Chapter11.indd
sea-level, a sailor sights a boat at an angle of depression of 27°. Calculate the horizontal distance of the boat from the sailor.
11:36:44 PM
Now
AB 5 AC 2 BC
5 (373.1 2 235.8) m
the beginning of the runway as 25°. Calculate the horizontal distance of the aircraft from the runway.
AB 5 137.3 m
Hence the distance between the two boats is 137.3 m.
595
[
545o
building acro angle of depr is 40° and the the building i same level gr (a) Calculate
base of th sured alo
53.5°. At what distance is the truck from the
65 10. From a coastal lookout point P, 100 m above 11/17/2009
B
B
238
238
238
238
B
B