Page 84 - Math SL HB Sem 3

(b) (i) A student is selected at random. Given that the student takes music, write down the
probability the student takes art.

(ii) Hence, show that taking music and taking an are not independent events.
(.t)

(c) Two sludents are selected at random. one after the other. Find the probability that the first
student takes only music and the second student takes only an.
(.1)
(Total l3 marks)

5. A company uses two machines. A and B, to make boxes. Machine A makes 60 % ofthe boxes.

80 % ofthe boxes made by machine A pass inspection.
90 o4 ofthe boxes made by machine B pass inspeclion.

A box is selected at random.

(a) Find the probabilitl that it passes inspection.
(3)

(b) The company would like the probability that a box passes inspecrion to be 0.87.
Find the percentage of boxes thal should be made by machine B ro achieve this.
(4)
(Total 7 marks)
6. In any given season, a soccer team plays 65 % oftheir games at home.
When the team plays at home, they win 83 % oftheir games.
When they play away from home, they win 26 % oftheir games.

The team plays one game.

(a) Find the probability tltat the team wins the game.
(l)

(b) Il'the team does not win the game. flnd the probability that the game was played at horre.
(1)
(Total 8 marks)

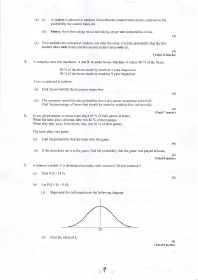
7. A random variable -l'is distributed normally with a mean of 20 and variance 9

(a) I.-ind P(-Y124.5).
(J)

(b) Let P(-Y< r) : 0.85.

(i) Represent this infomation on the follorving diagram.

t0
(ii) Find the value ofi.
(s)
(Total8 marks)

I