Page 277 - Six Sigma Advanced Tools for Black Belts and Master Black Belts

August 31, 2006
Char Count= 0
3:5
JWBK119-17
262 Strategies for Experimentation under Operational Constraints
17.5 INCOMPLETE EXPERIMENTAL DATA
The experimental design process could also encounter cases where no satisfactory
matrix can be constructed to exclude all physically infeasible conditions. Under such
circumstances, unless the experiment is abandoned altogether, the resulting data set
will not be complete. In certain other situations, the loss of essential measurements is
unexpected, owing to spoilage of samples or sudden equipment breakdown. Regard-
less of whether the missing data is foreseeable, it is still possible to extract whatever
information is available in the incomplete data set via an expedient known as ‘lean
design’, 11 making use of the effect sparsity principle: since not all effects generated
by the factors in an experiment are significant, one may depend on reduced degrees
of freedom in the data set to perform the necessary statistical analysis after some a
priori assignment of zero values to certain effects. In such an event, experience and
technical judgment will be extremely useful in determining which effects to ignore in
order to carry out a remedial data analysis.

17.6 ACCURACY OF LEAN DESIGN ANALYSIS

It is useful to note that the accuracy of the above analysis depends only on the validity
of the zero-value assumption about the selected effects, and is unrelated to the value of
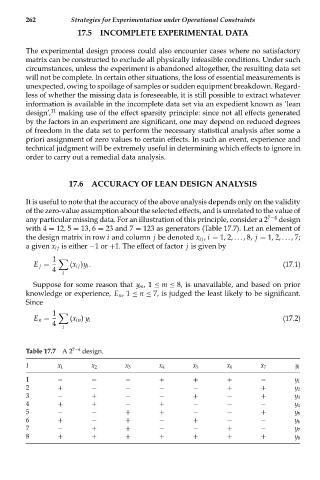
any particular missing data. For an illustration of this principle, consider a 2 7−4 design
with 4 = 12, 5 = 13, 6 = 23 and 7 = 123 as generators (Table 17.7). Let an element of
the design matrix in row i and column j be denoted x ij , i = 1, 2, . . . , 8, j = 1, 2, . . . , 7;
a given x ij is either −1or +1. The effect of factor j is given by
1
E j = (x ij )y i . (17.1)
4
i
Suppose for some reason that y m ,1 ≤ m ≤ 8, is unavailable, and based on prior
knowledge or experience, E n ,1 ≤ n ≤ 7, is judged the least likely to be significant.
Since
1
E n = (x in ) y i (17.2)
4
i

Table 17.7 A2 7−4 design.
I x 1 x 2 x 3 x 4 x 5 x 6 x 7 y i

1 − − − + + + − y 1
2 + − − − − + + y 2
3 − + − − + − + y 3
4 + + − + − − − y 4
5 − − + + − − + y 5
6 + − + − + − − y 6
7 − + + − − + − y 7
8 + + + + + + + y 8