Page 49 - Mathematics of Business and Finance

Chapter 1 | Review of Basic Arithmetic 29

Basic Arithmetic Operations with Fractions

When performing additions and subtractions of fractions, it is necessary to determine their equivalent
fractions using the least common denominator (LCD). When performing multiplications and divisions
of fractions, it is necessary to convert any mixed number to an improper fraction.

Adding Fractions
Addition of fractions requires that the denominators of every fraction be the same. To make them
the same:

Step 1: Determine the LCD.
Step 2: Convert each fraction to its equivalent fraction with the LCD as the common denominator.

Step 3: Add the numerators of the equivalent fractions, keeping the LCD as the denominator. (That
is, the resulting fraction will have the common denominator, and its numerator will be the
result of adding the numerators of the equivalent fractions.)
Express the final answer reduced to its lowest terms and as a mixed number, wherever applicable.

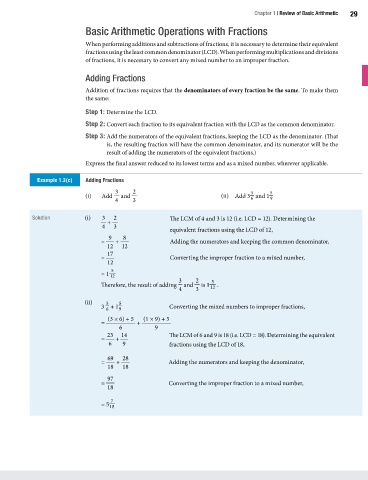
Example 1.3(c) Adding Fractions
3 2 5 5
(i) Add and (ii) Add 3 and 1
4 3 6 9

Solution (i) 3 2 The LCM of 4 and 3 is 12 (i.e. LCD = 12). Determining the

4 +
3
equivalent fractions using the LCD of 12,
9 8
= + Adding the numerators and keeping the common denominator,
12 12
17
= Converting the improper fraction to a mixed number,
12
5
= 1 12
3
2
5
Therefore, the result of adding and is 1 .
4 3 12
(ii) 5 5
3 + 1 Converting the mixed numbers to improper fractions,
9
6
(3 × 6) + 5 (1 × 9) + 5
= +
6 9
23 14 The LCM of 6 and 9 is 18 (i.e. LCD = 18). Determining the equivalent
= +
6 9 fractions using the LCD of 18,
69 28
= + Adding the numerators and keeping the denominator,
18 18

97
= Converting the improper fraction to a mixed number,
18
7
= 5 18