Page 57 - Mathematics of Business and Finance

Chapter 1 | Review of Basic Arithmetic 37

1
1
3
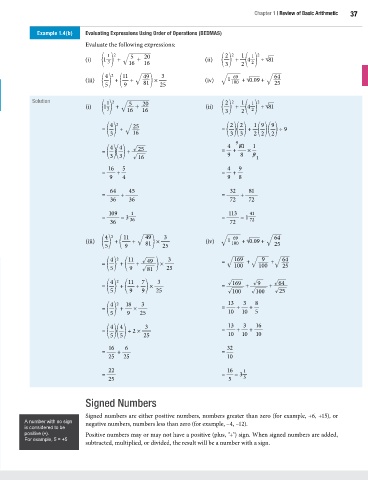
39. It took three consultants 27 hours, 21 hours, and 18 hours, respectively, to design a product. If each of them was Example 1.4(b) Evaluating Expressions Using Order of Operations (BEDMAS)
2
4
4
paid $55 per hour, what was the total amount paid to them? Evaluate the following expressions:
1
20
2 2
2
5
1 2 2
1
1
1
3
1
1
`
40. Three software programmers worked 17 hours, 25 hours, and 11 hours, respectively, to develop an e-commerce (i) e1 o + + 16 + 16 (ii) e o + e4 o ÷ √81
j
2
4
4
2
3 3
3
2
site. If each of them was paid $18 per hour, how much did they receive in total?
3 3
4 2 2
4
69
. 009
(iii) e o + e c 11 11 + + 49 m o × 25 (iv) 1 1 100 69 + + + √0.09 + 64 64
` j
#
+
. 009 ++
5
9
81
25
25
100
25
9
5
1.4 Order of Operations (BEDMAS) Solution
1
1 2 2
5
2
2 2
1
1
1
j
`
(i) e1 o + + 16 + 20 (ii) e o + e4 o ÷ √81
2
16
3
2
3 3
When arithmetic expressions contain multiple operations with brackets, exponents, divisions,
2
9
2
1 9
4 2 2
4
multiplications, additions, and subtractions, the arithmetic operation is performed in the following = e o + + 25 = e oe o + e oe o ÷ 9
`j
Order of arithmetic sequence: 3 3 16 3 3 2 2 2
operations: 9
1
4
4
4
Brackets 1. Perform all operations within the brackets. If there is more than one bracket, start with the = e oe o + 25 = + 81 ×
Exponents innermost bracket and move outwards to complete all the brackets. 3 3 16 9 8 9 1
Divisions 2. Perform operations with exponents and roots.
4
Multiplications = 16 + 5 = + 9
Additions 3. Perform the necessary divisions and multiplications in the order in which they appear from left to right. 9 4 9 8
Subtractions
4. Complete the operation by performing the necessary additions and subtractions in the order in
which they appear from left to right. = 64 + 45 = 32 + 81
The order of operations - Brackets, Exponents, Divisions, Multiplications, Additions, Subtractions - 36 36 72 72
can be remembered by the acronym BEDMAS. 109 1 113 41
= = 3 36 = = 1 72
Example 1.4(a) Computing Arithmetic Expressions by Following the Order of Operations (BEDMAS) 36 72
Compute the following arithmetic expressions: 4 2 2 11 11 49 3 3 69 69 64 64
4
. 009
` j
(iii) e o + e c 9 + 81 m o × 25 (iv) 1 1 100 + + + √0.09 + +
#
+
+
. 009 +
5
25
100
2
2
(i) (100 – 3 × 24) ÷ 2 + 4 × 3 (ii) 6 + 4 × 50 ÷ (8 – 3) – 1 (iii) 12 + 3 [(8 × 5) ÷ 5] – 7 + 2 5 9 25 25
9
64
169 69
4 2 11 49 3 = 1 169 . 009 + + + 64 64
. 009 +
10 100
Solution (i) (100 – 3 × 24) ÷ 2 + 4 × 3 = e o + e 9 + 81 o × 25 100 0 + + + + 100 25 25
25
5
= (100 – 3 × 24) ÷ 2 + 4 × 3 Perform multiplication within the brackets,
= (100 – 72) ÷ 2 + 4 × 3 Perform subtraction within the brackets, 4 2 11 7 3 = 169 + 9 + 64
= 28 ÷ 2 + 4 × 3 Perform division and multiplication from left to right, = e o + e 9 + o × 25 100 100 25
5
9
= 14 + 4 × 3
4 2
= 14 + 12 Perform addition, = e o + 18 × 3 = 13 + 3 + 8
= 26 5 9 25 10 10 5
(ii) 6 + 4 × 50 ÷ (8 – 3) – 1 4 4 3 13 3 16
2
= 6 + 4 × 50 ÷ (8 – 3) – 1 Perform the operation within the brackets, = e oe o + 2 × 25 = 10 + 10 + 10
2
5
5
2
= 6 + 4 × 50 ÷ (5) – 1 Perform the operation with the exponent,
= 6 + 4 × 50 ÷ 25 – 1 Perform division and multiplication from left to right, = 16 + 6 = 32
= 6 + 200 ÷ 25 – 1 25 25 10
= 6 + 8 – 1 Perform addition and subtraction from left to right,
= 14 – 1 = 22 = 16 = 3 1
= 13 25 5 5
2
(iii) 12 + 3 [(8 × 5) ÷ 5] – 7 + 2
2
= 12 + 3 [(8 × 5) ÷ 5] – 7 + 2 Perform the operation within the inner brackets, Signed Numbers
2
= 12 + 3 [40 ÷ 5] – 7 + 2 Perform the operation within the outer brackets, Signed numbers are either positive numbers, numbers greater than zero (for example, +6, +15), or
2
= 12 + 3 (8) – 7 + 2 Perform the operation with the exponent, A number with no sign negative numbers, numbers less than zero (for example, –4, –12).
is considered to be
= 12 + 9(8) – 7 + 2 Perform multiplication, positive (+). Positive numbers may or may not have a positive (plus, "+") sign. When signed numbers are added,
= 12 + 72 – 7 + 2 Perform addition and subtraction from left to right, For example, 5 = +5 subtracted, multiplied, or divided, the result will be a number with a sign.
= 84 – 7 + 2
= 77 + 2
= 79