Page 59 - Mathematics of Business and Finance

Chapter 1 | Review of Basic Arithmetic 39

Addition and Subtraction of Signed Numbers For example,
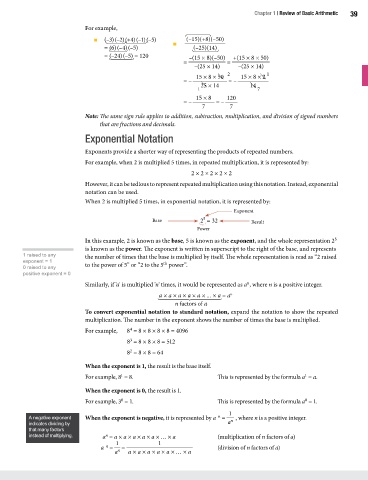
■ (–3) (–2) (+4) (–1) (–5) (–15)(+8)(–50)
The following are rules to be followed while adding or subtracting signed numbers. = (6) (–4) (–5) ■ (–25)(14)
Adding Two Signed Numbers = (–24) (–5) = 120 –(15 × 8)(–50) +(15 × 8 × 50)
a. If the signs of the two numbers are the same: disregard the sign of the numbers, add the numbers, = –(25 × 14) = –(25 × 14)
and keep the common sign. 15 × 8 × 50 2 15 × 8 × 2 1
For example, = – 25 × 14 = – 14
■ Adding +7 and +3 ■ Adding –6 and –2 1 7
= +7 + (+3) = –6 + (–2) = – 15 × 8 = – 120
7
7
= +(7 + 3) = –(6 + 2) Note: The same sign rule applies to addition, subtraction, multiplication, and division of signed numbers
= +10 = –8 that are fractions and decimals.
b. If the signs of the two numbers are different: disregard the sign of the numbers, subtract the smaller
Two signs should not be
written next to each other. number from the larger number, and keep the sign of the larger number. Exponential Notation
Brackets should be used to For example,
separate the two signs. Exponents provide a shorter way of representing the products of repeated numbers.
For example, we write ■ Adding +8 and –15 ■ Adding –3 and +7
–7 – (+2) instead of –7 – +2 = +8 + (–15) = –3 + (+7) For example, when 2 is multiplied 5 times, in repeated multiplication, it is represented by:
+8 + (–5) instead of +8 + –5 2 × 2 × 2 × 2 × 2
+10(–2) instead of +10 × –2 = –(15 – 8) = +(7 – 3)
= –7 = +4 However, it can be tedious to represent repeated multiplication using this notation. Instead, exponential
Subtracting Two Signed Numbers notation can be used.
When 2 is multiplied 5 times, in exponential notation, it is represented by:
Change the subtraction (minus) sign to addition (plus) and change the sign of the number being subtracted.
Then follow the above addition rules. Exponent
5
For example, Base 2 = 32 Result
■ Subtracting –12 from +18 ■ Subtracting –5 from –7 ■ Subtracting –6 from –2 Power
5
= +18 – (–12) = –7 – (–5) = –2 – (–6) In this example, 2 is known as the base, 5 is known as the exponent, and the whole representation 2
= +18 + (+12) = –7 + (+5) = –2 + (+6) 1 raised to any is known as the power. The exponent is written in superscript to the right of the base, and represents
= +(18 + 12) = –(7 – 5) = +(6 – 2) exponent = 1 the number of times that the base is multiplied by itself. The whole representation is read as “2 raised
th
= +30 = –2 = +4 0 raised to any to the power of 5” or “2 to the 5 power”.
positive exponent = 0
Multiplication and Division of Signed Numbers Similarly, if 'a' is multiplied 'n' times, it would be represented as a , where n is a positive integer.
n
The following are rules to be followed while multiplying or dividing two signed numbers: a × a × a × a × a × ... × a = a n
Multiplying two 1444444 2444444 3
n factors of a
signed numbers a. If the sign of the two numbers are the same: their product or quotient will be positive.
(+) (+) = (+) To convert exponential notation to standard notation, expand the notation to show the repeated
(−) (−) = (+) For example, multiplication. The number in the exponent shows the number of times the base is multiplied.
(+) (−) = (−)
4
(−) (+) = (−) ■ (+5)(+4) = +20 ■ (–5)(–4) = +20 For example, 8 = 8 × 8 × 8 × 8 = 4096
3
+12 –12 8 = 8 × 8 × 8 = 512
■ = +4 ■ = +4
+3 –3 8 = 8 × 8 = 64
2
b. If the sign of the two numbers are different: their product or quotient will be negative. When the exponent is 1, the result is the base itself.
For example,
1
Dividing two signed ■ (+5)(–4) = –20 ■ (–5)(+4) = –20 For example, 8 = 8. This is represented by the formula a = a.
1
numbers
+12 –12 When the exponent is 0, the result is 1.
(+) (–) ■ –3 = –4 ■ +3 = –4
0
(+) = (+) (–) = (+) For example, 3 = 1. This is represented by the formula a = 1.
0
(–) (+) ■ –25 = –5 or – 5 ■ +30 = +15 = –15 or – 15 1
–n
(+) = (–) (–) = (–) +15 3 3 –4 –2 2 2 A negative exponent When the exponent is negative, it is represented by a = n , where n is a positive integer.
indicates dividing by a
When multiplying or dividing more than two signed numbers, group them into pairs and determine that many factors
n
the sign using the rules for multiplication and division of signed numbers. instead of multiplying. a = a × a × a × a × a × … × a (multiplication of n factors of a)
1
1
–n
a = = (division of n factors of a)
a n a × a × a × a × a × … × a