Page 63 - Mathematics of Business and Finance

Chapter 1 | Review of Basic Arithmetic 43

5
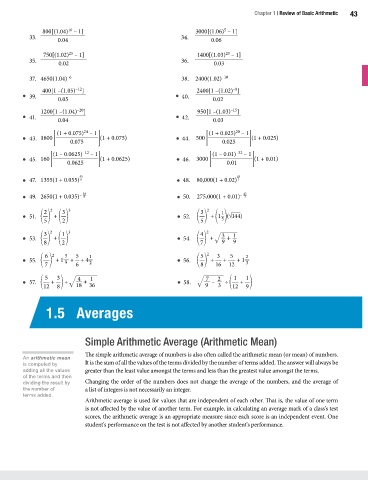
800[(1.04) – 1] 3000[(1.06) – 1]
10
33. 0.04 34. 0.06
25
20
750[(1.02) – 1] 1400[(1.03) – 1]
35. 0.02 36. 0.03
37. 4650(1.04) –6 38. 2400(1.02) –10
400[1 –(1.05) –12 ] 2400[1 –(1.02) ]
–8
39. 0.05 40. 0.02

1200[1 –(1.04) –20 ] 950[1 –(1.03) –15 ]
41. 0.04 42. 0.03

24
(1 + 0.075) – 1 (1 + 0.025) – 1
20
43. 1800 > H(1 + 0.075) 44. 500 > H(1 + 0.025)
0.075 0.025
(1 – 0.0625) –12 – 1 (1 – 0.01) –32 – 1
45. 160 > H(1 + 0.0625) 46. 3000 > H(1 + 0.01)
0.0625 0.01
55 43
47. 1355(1 + 0.055) 6 48. 80,000(1 + 0.02) 3

49. 2650(1 + 0.035) – 29 6 50. 275,000(1 + 0.01) – 43 3

2 2 3 3 3 2 1
51. e o + e o 52. e o + e 1 o(√144)
5
5 2 5
3 2 1 3 4 4 2 2 3 1
`j
53. e o + e o 54. e o + + +
8 2 7 7 9 9
6 2 5 5 1 5 2 3 5 2
55. e o + 1 + + 4 2 56. e o + + + 1 3
9
7 6 8 16 12
5 3 4 1 7 2 1 1
57. e + o ÷ + 58. – ÷ e + o
12 8 18 36 9 3 12 9

1.5 Averages

Simple Arithmetic Average (Arithmetic Mean)

The simple arithmetic average of numbers is also often called the arithmetic mean (or mean) of numbers.
An arithmetic mean
is computed by It is the sum of all the values of the terms divided by the number of terms added. The answer will always be
adding all the values greater than the least value amongst the terms and less than the greatest value amongst the terms.
of the terms and then
dividing the result by Changing the order of the numbers does not change the average of the numbers, and the average of
the number of a list of integers is not necessarily an integer.
terms added.
Arithmetic average is used for values that are independent of each other. That is, the value of one term
is not affected by the value of another term. For example, in calculating an average mark of a class's test
scores, the arithmetic average is an appropriate measure since each score is an independent event. One
student's performance on the test is not affected by another student's performance.