Page 95 - J. C. Turner "History and Science of Knots"

84 History and Science of Knots

the quipu.
AS120 AS143 AS149
b1 = 0.110
b2 = 0.228
b 0.340 0.338 0.222
Cl = 0.105
c2 = 0.534
C3 = 0.017
c 0.425 0.437 0.656
a 0.235 0.225 0.122
Naming the ratios from quipu AS120 a, b and c in the order of increasing
value, we find that they solve the equation
c-a c
b-a a

This relation was also studied in ancient Greece. But don't be tempted-we
cannot assume that there was any contact. If we add the first two values of
AS143, i.e. b1 and b2, and the middle three values of AS149, i.e. C1, c2 and c3,
we get three values per quipu which, named in the same order as in AS120, also
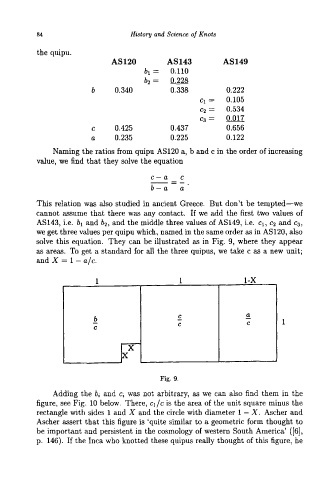
solve this equation. They can be illustrated as in Fig. 9, where they appear
as areas. To get a standard for all the three quipus, we take c as a new unit;
and X = 1 - a/c.

1-X

C a
b c 1
C C

Fig. 9.

Adding the bi and ci was not arbitrary, as we can also find them in the
figure, see Fig. 10 below. There, cl/c is the area of the unit square minus the
rectangle with sides 1 and X and the circle with diameter 1 - X. Ascher and
Ascher assert that this figure is `quite similar to a geometric form thought to
be important and persistent in the cosmology of western South America' (16],
p. 146). If the Inca who knotted these quipus really thought of this figure, he