Page 85 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 65

2.3.3 Boundary Conditions for Tangential Components of Field

The similar boundary conditions for tangential components of electric and magnetic fields can
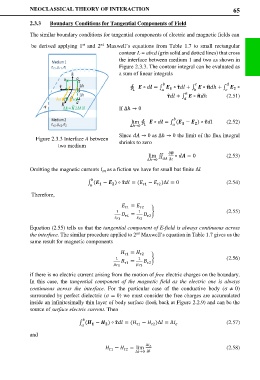
be derived applying 1 st and 2 nd Maxwell’s equations from Table 1.7 to small rectangular
contour L = abcd (grin solid and dotted lines) that cross
the interface between medium 1 and two as shown in
Figure 2.3.3. The contour integral can be evaluated as
a sum of linear integrals

∮ ∘ = ∫ ∘ � + ∫ ∘ �ℎ + ∫ ∘

� + ∫ ∘ �ℎ (2.51)

If ∆ℎ → 0

lim ∮ ∘ = ∫ ( − ) ∘ � (2.52)

∆ℎ→0
Since → 0 as ∆ℎ → 0 the limit of the flux integral
Figure 2.3.3 Interface A between shrinks to zero
two medium

lim ∬ ∘ = 0 (2.53)
∆ℎ→0
Omitting the magnetic currents as a fiction we have for small but finite ∆

∫ ( − ) ∘ � = ( − )∆ = 0 (2.54)

1 2
Therefore,

1 = 2
1 1 � (2.55)
1 = 2
1 2
Equation (2.55) tells us that the tangential component of E-field is always continuous across
the interface. The similar procedure applied to 2 Maxwell’s equation in Table 1.7 gives us the
nd
same result for magnetic components
1 = 2
1 1 � (2.56)
1 = 2
1 2
if there is no electric current arising from the motion of free electric charges on the boundary.
In this case, the tangential component of the magnetic field as the electric one is always
continuous across the interface. For the particular case of the conductive body ( ≠ 0)
surrounded by perfect dielectric ( = 0) we must consider the free charges are accumulated
inside an infinitesimally thin layer of body surface (look back at Figure 2.2.9) and can be the
source of surface electric current. Then

∫ ( − ) ∘ � = ( − )∆ = ∆ (2.57)

1 2
and

∆
− 2 = lim (2.58)
1
∆→0 ∆