Page 80 - Maxwell House

60 Chapter 2

amounts. It can be reached by varying sphere diameter, their number per unit volume and lattice
structure. Studies indicated that the same effect could be achieved if the metal microspheres are
replaced with layers of tiny metal disks, spherical
dielectric particles with high dielectric constant, metal
rods and strips, metal microspheres with dielectric
coatings, magnetodielectric spheres, and many other
components. The effective permittivity and
permeability of such a medium can be expressed in
terms of the electric and magnetic moments of the
spheres.

The idea how to develop and use such artificial
dielectric was proposed first in 1948 by American
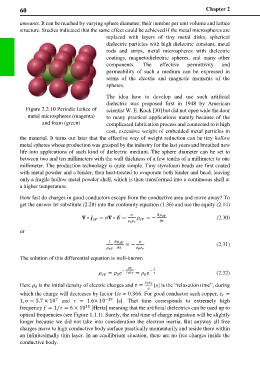
Figure 2.2.10 Periodic lattice of scientist W. E. Kock [30] but did not open wide the door
metal microspheres (magenta) to many practical applications mainly because of the
and foam (green) complicated fabrication process and connected to it high
cost, excessive weight of embedded metal particles in
the material. It turns out later that the effective way of weight reduction can be tiny hollow
metal spheres whose production was grasped by the industry for the last years and breathed new
life into applications of such kind of dielectric medium. The sphere diameter can be set to
between two and ten millimeters with the wall thickness of a few tenths of a millimeter to one
millimeter. The production technology is quite simple. Tiny styrofoam beads are first coated
with metal powder and a binder, then heat-treated to evaporate both binder and bead, leaving
only a fragile hollow metal powder shell, which is then transformed into a continuous shell at
a higher temperature.

How fast do charges in good conductors escape from the conductive area and move away? To
get the answer let substitute (2.28) into the continuity equation (1.36) and use the equity (2.14)

∘ = ∘ = = − (2.30)
0
or

1 (2.31)
= −
0
The solution of this differential equation is well-known

− −
= 0 = (2.32)
0
0
0
Here is the initial density of electric charges and = [s] is the “relaxation time”, during
0

which the charge will decreases by factor 1/e ≈ 0.366. For good conductor such copper, =

7
1, = 5.7 × 10 and = 1.6× 10 −19 [s]. That time corresponds to extremely high
frequency = 1 = 6 × 10 [Hertz] meaning that the artificial dielectrics can be used up to
18
⁄
optical frequencies (see Figure 1.1.1). Surely, the real time of charge migration will be slightly
longer because we did not take into consideration the electron inertia. But anyway all free
charges move to high conductive body surface practically momentarily and reside there within
an infinitesimally thin layer. In an equilibrium situation, there are no free charges inside the
conductive body.