Page 75 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 55

1/3
� � = ∑ ( ) 1/3 (2.16)

=1
Here and is the

volume fraction and
the relative dielectric
constant of each
constituent material,
respectively, N is the
number of different
components in
mixture. The equation
a) b)
(2.16) is some kind of
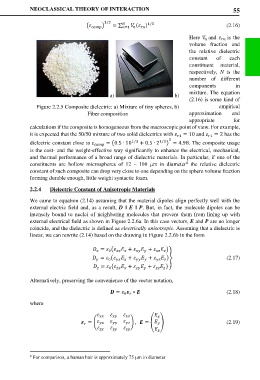
Figure 2.2.5 Composite dielectric: a) Mixture of tiny spheres, b) empirical
Fiber composition approximation and
appropriate for
calculations if the composite is homogeneous from the macroscopic point of view. For example,
it is expected that the 50/50 mixture of two solid dielectrics with 1 = 10 and 1 = 2 has the
3
⁄
⁄
dielectric constant close to = �0.5 ∙ 10 1 3 + 0.5 ∙ 2 1 3 � = 4.98. The composite usage
is the cost- and the weight-effective way significantly to enhance the electrical, mechanical,
and thermal performance of a broad range of dielectric materials. In particular, if one of the
8
constituents are hollow microspheres of 12 – 100 μm in diameter the relative dielectric
constant of such composite can drop very close to one depending on the sphere volume fraction
forming durable enough, little weight syntactic foam.
2.2.4 Dielectric Constant of Anisotropic Materials
We came to equation (2.14) assuming that the material dipoles align perfectly well with the
external electric field and, as a result, D || E || P. But, in fact, the molecule dipoles can be
intensely bound to nuclei of neighboring molecules that prevent them from lining up with
external electrical field as shown in Figure 2.2.6a. In this case vectors, E and P are no longer
coincide, and the dielectric is defined as electrically anisotropic. Assuming that a dielectric is
linear, we can rewrite (2.14) based on the drawing in Figure 2.2.6b in the form

= � + + �

0

= � + + �� (2.17)

0
= � + + �
0

Alternatively, preserving the convenience of the vector notation,
= ∘ (2.18)
0
where

� , = � � (2.19)
= �

8 For comparison, a human hair is approximately 75 μm in diameter