Page 73 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 53

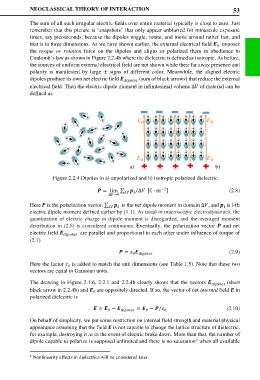
The sum of all such irregular electric fields over entire material typically is close to zero. Just
remember that this picture is "snapshots" that only appear unblurred for minuscule exposure
times, say picoseconds, because the dipoles wiggle, rotate, and move around rather fast, and
that is in three dimensions. As we have shown earlier, the external electrical field imposes
0
the torque or rotation force on the dipoles and aligns or polarized them in obedience to
Coulomb’s law as shown in Figure 2.2.4b where the dielectric is defined as isotropic. As before,
the sources of uniform external electrical field are not shown while their far away presence and
polarity is manifested by large ± signs of different color. Meanwhile, the aligned electric
dipoles produce its own net electric field (sum of black arrows) that reduce the external
electrical field. Then the electric dipole moment in infinitesimal volume Δ of material can be
defined as

a) b)
Figure 2.2.4 Dipoles in a) unpolarized and b) isotropic polarized dielectric

−2
⁄
= lim ∑ ∆ Δ [C ∙ m ] (2.8)

Δ→0
Here P is the polarization vector, ∑ ∆ is the net dipole moment in domain Δ, and is i-th

electric dipole moment defined earlier by (1.1). As usual in macroscopic electrodynamics, the
quantization of electric charge in dipole moment is disregarded, and the averaged moment
distribution in (2.8) is considered continuous. Eventually, the polarization vector and net
electric field are parallel and proportional to each other under influence of torque of
(2.7)
= (2.9)
0
Here the factor is added to match the unit dimensions (see Table 1.5). Note that these two
0
vectors are equal in Gaussian units.
The drawing in Figure 2.1.6, 2.2.1 and 2.2.4b clearly shows that the vectors (short
black arrow in 2.2.4b) and are oppositely directed. If so, the vector of net internal field in
0
polarized dielectric is

⁄
= − = − (2.10)
0
0
0
On behalf of simplicity, we put some restriction on internal field strength and material physical
appearance assuming that the field is not capable to change the lattice structure of dielectric,
for example, destroying it as in the event of electric brake dawn. More than that, the number of
dipole capable to polarize is supposed unlimited and there is no saturation when all available
4
4 Nonlinearity effects in dielectrics will be considered later.