Page 68 - Maxwell House

48 Chapter 2

product of twisting force value and the distance from the point of the force application. Since
the physical meaning of such product is the work or energy, the torque is equal to the kinetic
energy of rotation and measured in Joules. Let us recall some examples from mechanics. The
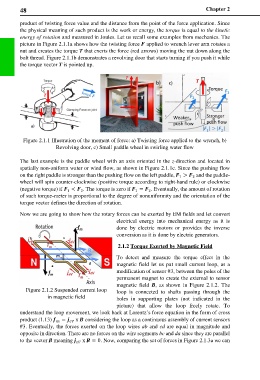
picture in Figure 2.1.1a shows how the twisting force F applied to wrench lever arm rotates a
nut and creates the torque T that exerts the force (red arrows) moving the nut down along the
bolt thread. Figure 2.1.1b demonstrates a revolving door that starts turning if you push it while
the torque vector T is pointed up.

Figure 2.1.1 Illustration of the moment of force: a) Twisting force applied to the wrench, b)
Revolving door, c) Small paddle wheel in swirling water flow

The last example is the paddle wheel with an axis oriented in the z-direction and located in
spatially non-uniform water or wind flow, as shown in Figure 2.1.1c. Since the pushing flow
on the right paddle is stronger than the pushing flow on the left paddle, > and the paddle-
1
2
wheel will spin counter-clockwise (positive torque according to right-hand rule) or clockwise
(negative torque) if < . The torque is zero if = . Eventually, the amount of rotation
1
2
1
2
of such torque-meter is proportional to the degree of nonuniformity and the orientation of the
torque vector defines the direction of rotation.
Now we are going to show how the rotary forces can be exerted by EM fields and let convert
electrical energy into mechanical energy as it is
done by electric motors or provides the inverse
conversion as it is done by electric generators.
2.1.2 Torque Exerted by Magnetic Field

To detect and measure the torque effect in the
magnetic field let us put small current loop, as a
modification of sensor #3, between the poles of the
permanent magnet to create the external to sensor
magnetic field B, as shown in Figure 2.1.2. The
Figure 2.1.2 Suspended current loop loop is connected to shafts passing through the
in magnetic field holes in supporting plates (not indicated in the
picture) that allow the loop freely rotate. To
understand the loop movement, we look back at Lorentz’s force equation in the form of cross
product (1.13) = x considering the loop as a continuous assembly of current sensors

#3. Eventually, the forces exerted on the loop wires ab and cd are equal in magnitude and
opposite in direction. There are no forces on the wire segments bc and da since they are parallel
to the vector B meaning x = 0. Now, comparing the set of forces in Figure 2.1.3a we can