Page 71 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 51

2.1.3 Torque Exerted by Electric Field

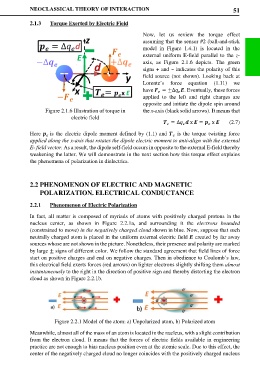
Now, let us review the torque effect
assuming that the sensor #2 (ball-and-stick
model in Figure 1.4.1) is located in the
external uniform E-field parallel to the z-
axis, as Figure 2.1.6 depicts. The green
signs + and – indicates the polarity of this
field source (not shown). Looking back at
Lorentz’s force equation (1.11) we
have = ±Δ . Eventually, these forces

applied to the left and right charges are
opposite and initiate the dipole spin around
Figure 2.1.6 Illustration of torque in the x-axis (black solid arrows). It means that
electric field
= Δ x = x (2.7)

Here is the electric dipole moment defined by (1.1) and is the torque twisting force

applied along the x-axis that rotates the dipole electric moment to anti-align with the external
E- field vector. As a result, the dipole self-field occurs in opposite to the external E-field thereby
weakening the latter. We will demonstrate in the next section how this torque effect explains
the phenomena of polarization in dielectrics.

2.2 PHENOMENON OF ELECTRIC AND MAGNETIC
POLARIZATION. ELECTRICAL CONDUCTANCE

2.2.1 Phenomenon of Electric Polarization

In fact, all matter is composed of myriads of atoms with positively charged protons in the
nucleus center, as shown in Figure 2.2.1a, and surrounding it the electrons bounded
(constrained to move) in the negatively charged cloud shown in blue. Now, suppose that such
neutrally charged atom is placed in the uniform external electric field E created by far away
sources whose are not shown in the picture. Nonetheless, their presence and polarity are marked
by large ± signs of different color. We follow the standard agreement that field lines of force
start on positive charges and end on negative charges. Then in obedience to Coulomb’s law,
this electrical field exerts forces (red arrows) on lighter electrons slightly shifting them almost
instantaneously to the right in the direction of positive sign and thereby distorting the electron
cloud as shown in Figure 2.2.1b.

Figure 2.2.1 Model of the atom: a) Unpolarized atom, b) Polarized atom
Meanwhile, almost all of the mass of an atom is located in the nucleus, with a slight contribution
from the electron cloud. It means that the forces of electric fields available in engineering
practice are not enough to bias nucleus position even at the atomic scale. Due to this effect, the
center of the negatively charged cloud no longer coincides with the positively charged nucleus