Page 76 - Maxwell House

56 Chapter 2

The matrix is

called the relative
dielectric tensor and
the equity (2.19)
reflects the simple
fact that the electric
field polarizes the
anisotropic dielectric
a) b) more easily in some
directions than in
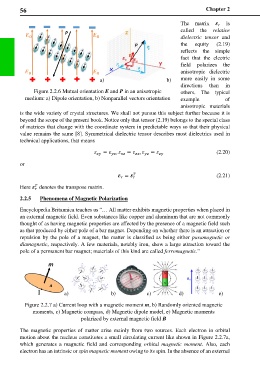
Figure 2.2.6 Mutual orientation E and P in an anisotropic others. The typical
medium: a) Dipole orientation, b) Nonparallel vectors orientation example of
anisotropic materials
is the wide variety of crystal structures. We shall not pursue this subject further because it is
beyond the scope of the present book. Notice only that tensor (2.19) belongs to the special class
of matrices that change with the coordinate system in predictable ways so that their physical
value remains the same [8]. Symmetrical dielectric tensor describes most dielectrics used in
technical applications, that means

= , = , = (2.20)

or
= (2.21)

Here denotes the transpose matrix.

2.2.5 Phenomena of Magnetic Polarization
Encyclopedia Britannica teaches us “… All matter exhibits magnetic properties when placed in
an external magnetic field. Even substances like copper and aluminum that are not commonly
thought of as having magnetic properties are affected by the presence of a magnetic field such
as that produced by either pole of a bar magnet. Depending on whether there is an attraction or
repulsion by the pole of a magnet, the matter is classified as being either paramagnetic or
diamagnetic, respectively. A few materials, notably iron, show a large attraction toward the
pole of a permanent bar magnet; materials of this kind are called ferromagnetic.”

a) b) c) d) e)

Figure 2.2.7 a) Current loop with a magnetic moment m, b) Randomly oriented magnetic
moments, c) Magnetic compass, d) Magnetic dipole model, e) Magnetic moments
polarized by external magnetic field B
The magnetic properties of matter arise mainly from two sources. Each electron in orbital
motion about the nucleus constitutes a small circulating current like shown in Figure 2.2.7a,
which generates a magnetic field and corresponding orbital magnetic moment. Also, each
electron has an intrinsic or spin magnetic moment owing to its spin. In the absence of an external