Page 90 - Mechatronics with Experiments

76 MECHATRONICS
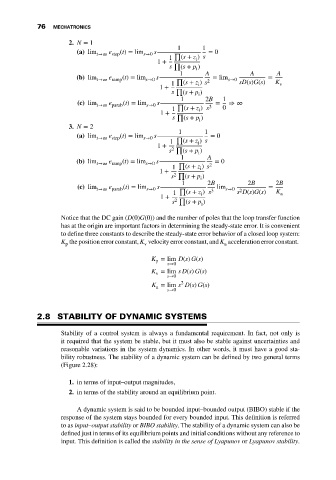
2. N = 1
1 1
e
(a) lim t→∞ step (t) = lim s→0 s ∏ = 0
1 (s + z ) s
i
1 + ∏
s (s + p )
i
1 A A A
e
(b) lim t→∞ ramp (t) = lim s→0 s ∏ = lim s→0 =
1 (s + z ) s 2 sD(s)G(s) K v
i
1 + ∏
s (s + p )
i
1 2B 1
(c) lim e (t) = lim s = ⇒ ∞
t→∞ parab s→0 ∏ 3
1 (s + z ) s 0
i
1 + ∏
s (s + p )
i
3. N = 2
1 1
e
(a) lim t→∞ step (t) = lim s→0 s ∏ = 0
1 (s + z ) s
i
1 + ∏
s 2 (s + p )
i
1 A
(b) lim t→∞ ramp (t) = lim s→0 s ∏ = 0
e
1 (s + z ) s 2
i
1 + ∏
s 2 (s + p )
i
1 2B 2B 2B
(c) lim e (t) = lim s lim =
t→∞ parab s→0 ∏ 3 s→0 2
1 (s + z ) s s D(s)G(s) K a
i
1 + ∏
s 2 (s + p )
i
Notice that the DC gain (D(0)G(0)) and the number of poles that the loop transfer function
has at the origin are important factors in determining the steady-state error. It is convenient
to define three constants to describe the steady-state error behavior of a closed loop system:
K the position error constant, K velocity error constant, and K acceleration error constant.
v
a
p
K = lim D(s) G(s)
p
s→0
K = lim sD(s) G(s)
v
s→0
2
K = lim s D(s) G(s)
a
s→0
2.8 STABILITY OF DYNAMIC SYSTEMS
Stability of a control system is always a fundamental requirement. In fact, not only is
it required that the system be stable, but it must also be stable against uncertainties and
reasonable variations in the system dynamics. In other words, it must have a good sta-
bility robustness. The stability of a dynamic system can be defined by two general terms
(Figure 2.28):
1. in terms of input–output magnitudes,
2. in terms of the stability around an equilibrium point.
A dynamic system is said to be bounded input–bounded output (BIBO) stable if the
response of the system stays bounded for every bounded input. This definition is referred
to as input–output stability or BIBO stability. The stability of a dynamic system can also be
defined just in terms of its equilibrium points and initial conditions without any reference to
input. This definition is called the stability in the sense of Lyapunov or Lyapunov stability.