Page 175 - classs 6 a_Neat

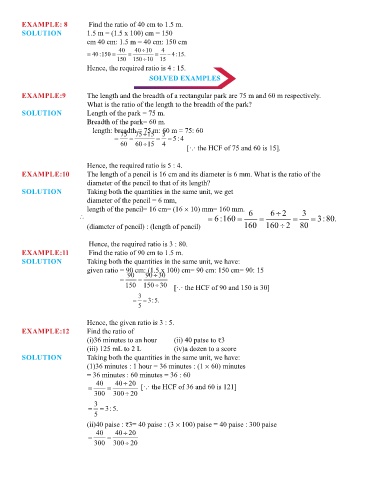
EXAMPLE: 8 Find the ratio of 40 cm to 1.5 m.
12 Ratio, Proportion And SOLUTION 1.5 m = (1.5 x 100) cm = 150

cm 40 cm: 1.5 m = 40 cm: 150 cm

Unitary Method
÷
= 40:150 = 40 = 40 10 = 4 − 4:15.
÷
a 150 150 10 15
RATIO OF NUMBERS The ratio of two nonzero numbers a and bis thefraction and we write it as a : b Hence, the required ratio is 4 : 15.
read as ‘a is to b’. b SOLVED EXAMPLES
In the ratio a : b we call a the first term or antecedent and b the second term or consequent. EXAMPLE:9 The length and the breadth of a rectangular park are 75 m and 60 m respectively.
EXAMPLE:1 3: 5 is a ratio in which first term is 3 and the second term is 5 and we define 3: 5 as 3 What is the ratio of the length to the breadth of the park?
5 SOLUTION Length of the park = 75 m.

AN IMPORTANT RESULT We know that a fraction does not change when its numerator and denominator are Breadth of the park= 60 m.
∴
multiplied or divided by the same nonzero number. So, a ratio does not change when its first and second terms length: breadth = 75 m: 60 m = 75: 60
÷
are multiplied or divided by the same nonzero number. = 75 = 75 15 = 5 = 5: 4
÷
2 24 8 60 60 15 4 [·.· the HCF of 75 and 60 is 15].
×
EXAMPLE:2 ( ) i 2:3= 3 = 3 4 = 12 = 8:12.
×
60 60 5 12 Hence, the required ratio is 5 : 4.
÷
( ) ii 60: 45 = = = = 12:9
÷
45 45 5 9 EXAMPLE:10 The length of a pencil is 16 cm and its diameter is 6 mm. What is the ratio of the
diameter of the pencil to that of its length?
EQUIVALENT RATIOS On multiplying (or dividing} each term of a ratio by the same non zero number we SOLUTION Taking both the quantities in the same unit, we get
get a ratio equivalent to the given ratio. diameter of the pencil = 6 mm,
EXAMPLE:3 (i) As shown above, 2: 3 and 8: 12 are equivalent ratios. length of the pencil= 16 cm= (16 × 10) mm= 160 mm.
÷
(ii) As shown above, 60 : 45 and 12 : 9 are equivalent ratios. ∴ = 6:160 = 6 = 62 = 3 = 3:80.
÷
(diameter of pencil) : (length of pencil) 160 160 2 80
RATIO IN SIMPLEST FORM The ratio a : b is said to be in the simplestform if the HCF of a and b is 1.
EXAMPLE:4 (i) The ratio 11 : 15 is in the simplest form since the HCF of 11 and 15 is 1. Hence, the required ratio is 3 : 80.
(ii) The ratio 15 : 20 is not in the simplest form since the HCF of 15 and 20 is 5, not 1. EXAMPLE:11 Find the ratio of 90 cm to 1.5 m.
SOLUTION Taking both the quantities in the same unit, we have:
TO CONVERT A GIVEN RATIO TO ITS SIMPLEST FORM To convert a given ratio a : b to its given ratio = 90 cm: (1.5 x 100) cm= 90 cm: 150 cm= 90: 15
÷
simplest form, we divide each term by the HCF of a and b. = 90 = 90 30
÷
EXAMPLE:5 Convert the ratio 40 : 25 in its simplest form. 150 150 30 [·.· the HCF of 90 and 150 is 30]

SOLUTION HCF of 40 and 25 is 5. 3
= = 3:5.
40 40 8 8 5
÷
∴ 40: 25 = = = = 8:5
25 25 5 5 Hence, the given ratio is 3 : 5.
÷
Hence, the simplest form of 40: 25 is 8 : 5. EXAMPLE:12 Find the ratio of
EXAMPLE:6 Express the ratio 87 : 58 in simplest form. (i)36 minutes to an hour (ii) 40 patse to ₹3
SOLUTION HCF of 87 and 58 is 29. (iii) 125 mL to 2 L (iv)a dozen to a score
87 87 29 3
÷
∴ 87 :58 = = = = 3: 2 SOLUTION Taking both the quantities in the same unit, we have:
÷
58 58 29 2 (1)36 minutes : 1 hour = 36 minutes : (1 × 60) minutes
= 36 minutes : 60 minutes = 36 : 60
RATIO OF TWO QUANTITIES IN SAME UNITS 40 40 20
÷
NOTE The ratio of two quantities is defined only when they are in the same unit. = 300 = 300 20 [·.· the HCF of 36 and 60 is 121]
÷
EXAMPIE:7 Suppose that Kunal’s weight is 28 kg and Tanvy’s weight is 32 kg. Then,
(Kunal’s weight) : (Tanvy’s weight) = 28 kg : 32 kg= 28 : 32 = 3 = 3:5.
5
÷
= 28 = 28 4 = 7 = 7 :8 (ii)40 paise : ₹3= 40 paise : (3 × 100) paise = 40 paise : 300 paise
32 32 4 8 40 40 20
÷
÷
∴
ratio of Kunal’s weight to Tanvy’s weight is 7 : 8. = 300 = 300 20
÷