Page 176 - classs 6 a_Neat

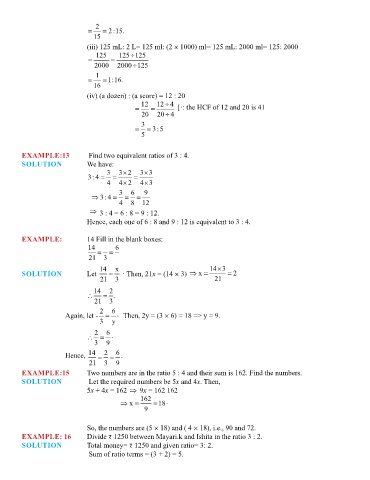
2  3    3
= = 2:15. Mayank’s share =  of `1250  = ` 1250 ×  = ⋅ ` 750

15  5    5
(iii) 125 mL: 2 L= 125 ml: (2 × 1000) ml= 125 mL: 2000 ml= 125: 2000  2    2
125 125 125 Ishita’s share =  of `1250  = ` 1250 ×  = ⋅ ` 500
÷

= =  5    5
÷
2000 2000 125 Example:17 Divide ₹1200 among A. B and C in the ratio 2 : 3 : 5.
1 SOLUTION Total money= t1200.
= = 1:16.
16 Sum of ratio terms= (2 + 3 + 5) = 10.
(iv) (a dozen) : (a score) = 12 : 20 Sum of ratio terms= (2 + 3 + 5) = 10.
12 12 4  2 
÷
= = [·: the HCF of 12 and 20 is 41 A’s share = ₹ 1200×  = ₹ 240.

20 20 4  10 
÷
3  3 
= = 3:5 1200× = ₹ 360.
5 B’s share = ₹  10  

EXAMPLE:13 Find two equivalent ratios of 3 : 4. C’s share = ₹ 1200× 5   = ₹ 600.

SOLUTION We have:  10 
3 3 2 33 COMPARISON OF RATIOS
×
×
3: 4 = = = Suppose we want to compare two given ratios. Then. we express each one of them as a fraction in the simplest
4 4 2 4 3
×
×
3 6 9 form. Now, compare these fractions by making their denominators equal.
⇒ 3: 4 = = =
4 8 12 Example:18 Compare the ratios (5: 6) and (3: 4).
⇒ 3 : 4 = 6 : 8 = 9 : 12. SOLUTION We can write:
Hence, each one of 6 : 8 and 9 : 12 is equivalent to 3 : 4. 5 3
)
)
(5:6 = 6 and (3: 4 = 4 ⋅
EXAMPLE: 14 Fill in the blank boxes: 5 3
14 = = 6 Now, let us compare and ⋅
21 3 6 4
×
14 x 14 3 The LCM of 6 and 4 is 12.
SOLUTION Let = · Then, 21x = (14 × 3) ⇒ x = = 2 Making the denominator of each fraction equal to 12, we have:
21 3 21 5 5 2 10 3 3 3 9
×
×
14 2 6 = 6 2 = 12 and 4 = 4 3 = 12 ⋅
×
×
∴ = .
21 3 Clearly, 10 > 9 ⇒ 5 <⋅
3
2 6 12 12 6 4
Again, let - = ⋅ Then, 2y = (3 × 6) = 18 => y = 9.
3 y Hence, (5 : 6) > (3 : 4).
2 6 Example:19 The ratio of copper and zinc in an alloy is 5 : 3. if the weight of copper in the alloy
∴= ⋅ is 30.5 g,Jind the weight of zinc in it.
3 9 Solution Ratio of copper and zinc in the alloy = 5 : 3.
6
Hence, 14 = 2 = ⋅ Let the weight of copper and zinc in it be (5×) g and (3×) g respectively.
21 3 9 Now, weight of copper = (30.5) g (given).
EXAMPLE:15 Two numbers are in the ratio 5 : 4 and their sum is 162. Find the numbers.
SOLUTION Let the required numbers be 5x and 4x. Then, ∴ 5x = 30.5 ⇒ x = 30.5 = 6.1.
5x + 4x = 162 ⇒ 9x = 162 162 5
162
⇒ x = = 18 ⋅
9 ∴ weight of zinc ( )g= 3x = (3 6.1 g 18.3g.× ) =

So, the numbers are (5 × 18) and ( 4 × 18), i.e., 90 and 72. Just try:1 Anuj has 5 kites and T anuj has 10 kites. Who has more kites?
EXAMPLE: 16 Divide ₹ 1250 between Mayari.k and Ishita in the ratio 3 : 2. Just try:2 Rohit is 3 years old. His brother Mohit is 6 years old. Compare their ages.
SOLUTION Total money= ₹ 1250 and given ratio= 3: 2. Just try:3 The time taken by Gopi to reach school is 1 hour and the time taken by Gunjan to reach school is 40
Sum of ratio terms = (3 + 2) = 5. minutes. Compare the time taken by the two students to reach school.