Page 36 - Nature Of Space And Time

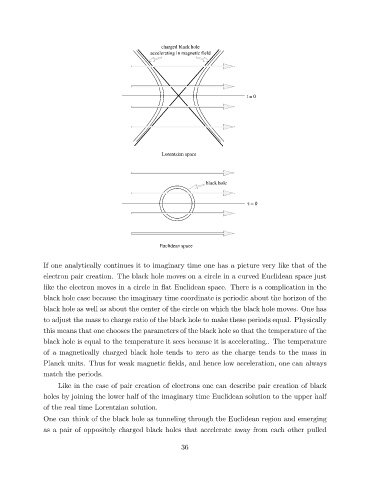
charged black hole
accelerating in magnetic field

t = 0

Lorentzian space

black hole

t = 0

Euclidean space

If one analytically continues it to imaginary time one has a picture very like that of the
electron pair creation. The black hole moves on a circle in a curved Euclidean space just

like the electron moves in a circle in
at Euclidean space. There is a complication in the
black hole case because the imaginary time coordinate is periodic about the horizon of the
black hole as well as about the center of the circle on which the black hole moves. One has
to adjust the mass to charge ratio of the black hole to make these periods equal. Physically

this means that one chooses the parameters of the black hole so that the temperature of the
black hole is equal to the temperature it sees because it is accelerating.. The temperature
of a magnetically charged black hole tends to zero as the charge tends to the mass in
Planck units. Thus for weak magnetic elds, and hence low acceleration, one can always

match the periods.
Like in the case of pair creation of electrons one can describe pair creation of black
holes by joining the lower half of the imaginary time Euclidean solution to the upper half

of the real time Lorentzian solution.
One can think of the black hole as tunneling through the Euclidean region and emerging
as a pair of oppositely charged black holes that accelerate away from each other pulled

36