Page 32 - Nature Of Space And Time

1
the S corresponds to the imaginary time direction which is identi ed periodically. One
can ll in this boundary with metrics of at least two di erent topologies. One of course
2
2
is the Euclidean-Schwarzschild metric. This has topology R × S , that is the Euclidean
1
3
two plane times a two sphere. The other is R × S , the topology of Euclidean
at space
periodically identi ed in the imaginary time direction. These two topologies have di erent
Euler numbers. The Euler number of periodically identi ed
at space is zero, while that
of the Euclidean-Schwarzschild solution is two.

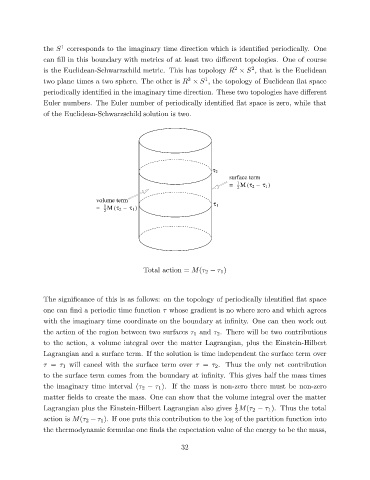
t 2
surface term
= 1 M(t 2 _ t 1 )
2
volume term
= 1 M(t 2 _ t 1 ) t 1
2

Total action = M( 2 − 1)

The signi cance of this is as follows: on the topology of periodically identi ed
at space
one can nd a periodic time function whose gradient is no where zero and which agrees

with the imaginary time coordinate on the boundary at in nity. One can then work out
the action of the region between two surfaces 1 and 2. There will be two contributions
to the action, a volume integral over the matter Lagrangian, plus the Einstein-Hilbert
Lagrangian and a surface term. If the solution is time independent the surface term over

= 1 will cancel with the surface term over = 2. Thus the only net contribution
to the surface term comes from the boundary at in nity. This gives half the mass times
the imaginary time interval ( 2 − 1). If the mass is non-zero there must be non-zero
matter elds to create the mass. One can show that the volume integral over the matter
1
Lagrangian plus the Einstein-Hilbert Lagrangian also gives M( 2 − 1). Thus the total
2
action is M( 2 − 1). If one puts this contribution to the log of the partition function into
the thermodynamic formulae one nds the expectation value of the energy to be the mass,

32