Page 27 - Nature Of Space And Time

r=0 singularity I +

4
+
r=constant
3 1 I 0

r = 2M

2 _

_
r=0 singularity I
future event horizon past event horizon

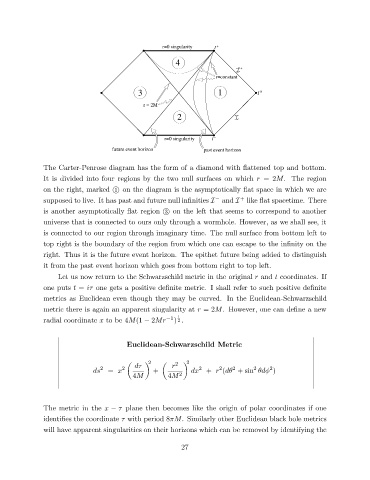
The Carter-Penrose diagram has the form of a diamond with
attened top and bottom.
It is divided into four regions by the two null surfaces on which r =2M. The region
on the right, marked
on the diagram is the asymptotically
at space in which we are
1
+
−
supposed to live. It has past and future null in nities I and I like
at spacetime. There
is another asymptotically
at region
on the left that seems to correspond to another
3
universe that is connected to ours only through a wormhole. However, as we shall see, it

is connected to our region through imaginary time. The null surface from bottom left to
top right is the boundary of the region from which one can escape to the in nity on the
right. Thus it is the future event horizon. The epithet future being added to distinguish
it from the past event horizon which goes from bottom right to top left.
Let us now return to the Schwarzschild metric in the original r and t coordinates. If

one puts t = i one gets a positive de nite metric. I shall refer to such positive de nite
metrics as Euclidean even though they may be curved. In the Euclidean-Schwarzschild
metric there is again an apparent singularity at r =2M. However, one can de ne a new
1
radial coordinate x to be 4M(1 − 2Mr −1 ) 2 .

Euclidean-Schwarzschild Metric

2 2 2
d r
2
2
2
2
2
ds 2 = x 2 + dx + r (d +sin d )
4M 4M 2
The metric in the x − plane then becomes like the origin of polar coordinates if one
identi es the coordinate with period 8 M. Similarly other Euclidean black hole metrics
will have apparent singularities on their horizons which can be removed by identifying the

27