Page 25 - Nature Of Space And Time

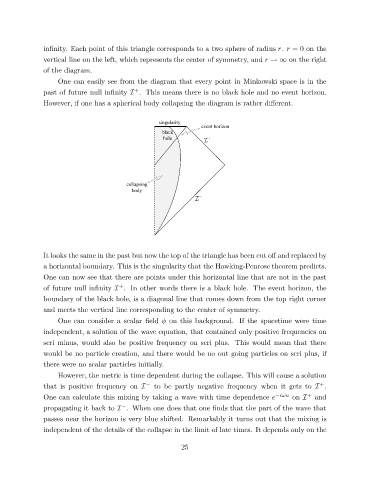
in nity. Each point of this triangle corresponds to a two sphere of radius r. r = 0 on the
vertical line on the left, which represents the center of symmetry, and r →∞ on the right
of the diagram.
One can easily see from the diagram that every point in Minkowski space is in the
+
past of future null in nity I . This means there is no black hole and no event horizon.
However, if one has a spherical body collapsing the diagram is rather di erent.

singularity
event horizon
black
hole +

collapsing
body
_

It looks the same in the past but now the top of the triangle has been cut o and replaced by
a horizontal boundary. This is the singularity that the Hawking-Penrose theorem predicts.

One can now see that there are points under this horizontal line that are not in the past
+
of future null in nity I . In other words there is a black hole. The event horizon, the
boundary of the black hole, is a diagonal line that comes down from the top right corner
and meets the vertical line corresponding to the center of symmetry.

One can consider a scalar eld on this background. If the spacetime were time
independent, a solution of the wave equation, that contained only positive frequencies on
scri minus, would also be positive frequency on scri plus. This would mean that there
would be no particle creation, and there would be no out going particles on scri plus, if

there were no scalar particles initially.
However, the metric is time dependent during the collapse. This will cause a solution
− +
that is positive frequency on I to be partly negative frequency when it gets to I .
One can calculate this mixing by taking a wave with time dependence e −i!u on I + and

propagating it back to I . When one does that one nds that the part of the wave that
−
passes near the horizon is very blue shifted. Remarkably it turns out that the mixing is
independent of the details of the collapse in the limit of late times. It depends only on the

25