Page 28 - Nature Of Space And Time

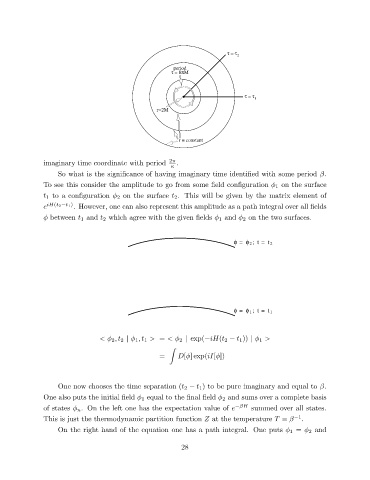
t = t
2
period
t = 8pM

t = t
1
r=2M

r = constant

imaginary time coordinate with period 2 .

So what is the signi cance of having imaginary time identi ed with some period .

To see this consider the amplitude to go from some eld con guration 1 on the surface
t 1 to a con guration 2 on the surface t 2. This will be given by the matrix element of
e iH(t 2 −t 1 ) . However, one can also represent this amplitude as a path integral over all elds
between t 1 and t 2 which agree with the given elds 1 and 2 on the two surfaces.

f= f 2 ;t = t 2

f= f 1 ;t = t 1

< 2;t 2 | 1;t 1 > = < 2 | exp(−iH(t 2 − t 1)) | 1 >

Z
= D[ ]exp(iI[ ])

One now chooses the time separation (t 2 − t 1) to be pure imaginary and equal to .
One also puts the initial eld 1 equal to the nal eld 2 and sums over a complete basis

of states n. On the left one has the expectation value of e − H summed over all states.
This is just the thermodynamic partition function Z at the temperature T = −1 .
On the right hand of the equation one has a path integral. One puts 1 = 2 and

28