Page 33 - Nature Of Space And Time

as one would expect. However, the entropy contributed by the background eld will be
zero.
The situation is di erent however with the Euclidean-Schwarzschild solution.

t= t 2
surface term
volume term = 0 1
= M(t 2 _ t 1 )
2
fixed two
sphere
t= t 1
r = 2M
surface term from corner
= 1 M(t 2 _ t 1 )
2

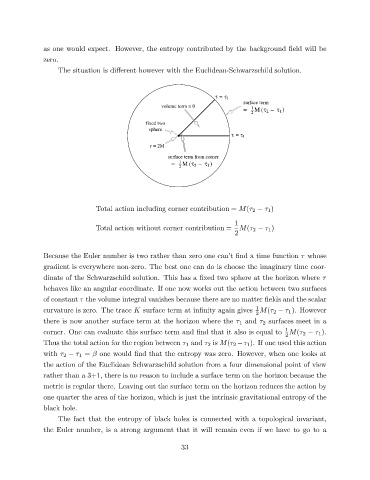
Total action including corner contribution = M( 2 − 1)

1
Total action without corner contribution = M( 2 − 1)
2

Because the Euler number is two rather than zero one can't nd a time function whose
gradient is everywhere non-zero. The best one can do is choose the imaginary time coor-

dinate of the Schwarzschild solution. This has a xed two sphere at the horizon where
behaves like an angular coordinate. If one now works out the action between two surfaces
of constant the volume integral vanishes because there are no matter elds and the scalar
1
curvature is zero. The trace K surface term at in nity again gives M( 2 − 1 ). However
2
there is now another surface term at the horizon where the 1 and 2 surfaces meet in a
1
corner. One can evaluate this surface term and nd that it also is equal to M( 2 − 1).
2
Thus the total action for the region between 1 and 2 is M( 2 − 1). If one used this action
with 2 − 1 = one would nd that the entropy was zero. However, when one looks at

the action of the Euclidean Schwarzschild solution from a four dimensional point of view
rather than a 3+1, there is no reason to include a surface term on the horizon because the
metric is regular there. Leaving out the surface term on the horizon reduces the action by
one quarter the area of the horizon, which is just the intrinsic gravitational entropy of the

black hole.
The fact that the entropy of black holes is connected with a topological invariant,
the Euler number, is a strong argument that it will remain even if we have to go to a

33