Page 44 - Nature Of Space And Time

+
M

S

_
M

Z
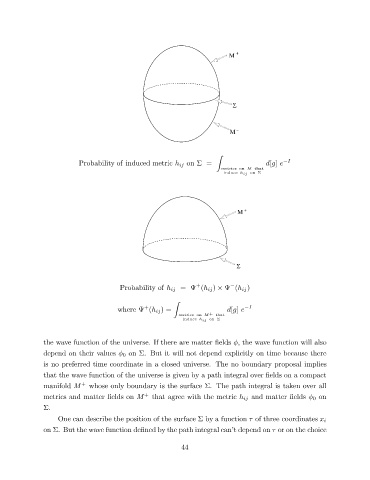
Probability of induced metric h ij on = d[g] e −I
metrics on M that
induce h ij on

M +

S

+
−
Probability of h ij = (h ij ) × (h ij )
Z
+
where (h ij )= d[g] e −I
metrics on M + that
induce h ij on

the wave function of the universe. If there are matter elds , the wave function will also
depend on their values 0 on . But it will not depend explicitly on time because there
is no preferred time coordinate in a closed universe. The no boundary proposal implies

that the wave function of the universe is given by a path integral over elds on a compact
manifold M + whose only boundary is the surface . The path integral is taken over all
metrics and matter elds on M + that agree with the metric h ij and matter elds 0 on

.
One can describe the position of the surface by a function of three coordinates x i
on . But the wave function de ned by the path integral can't depend on or on the choice

44