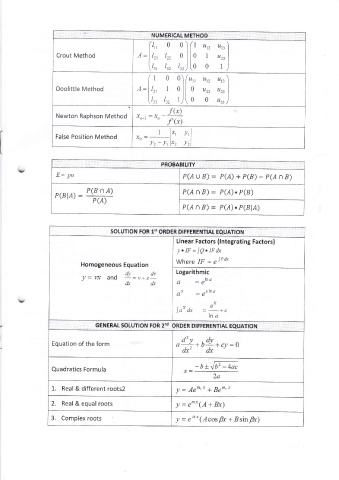
Page 2 - FORMULA DBM30043

NUMEBICAL METIIOD
(t,, 0 o) 1 ,r, 2,. \
tJ I
Crout Method ,q =l t-. tD ol 01 urr
I lt l
I t' lr, 00 t)
U,, )
(t 0 0) (r,, urz a,, )
I
ll
Doolittle Method A =l l^, 1 o o ,,, u,,l
| ''
U,, t"" rjlo o u,)
_f(*)
Newton Raphson Method Xr*l = Xn
f'(*)

1 x1 !r
False Position Method --
^o
lz-lr x2 !z

PROBABITITY
v
E= pn P(A u B) = P(A) + P(B) P(A n B)
-
P(B N A\ P(A n B) = P(A). P(B)
\''
P(BIA)
P(A)
P(A n B) = P(A). P(BIA)

SOLUTION FOR 1SI OfiDER DIffE*ENTIAL EQUATI.ON
Linear Factors (lntegrating Factors)
y. IF - lQ. tt'd*

Where IF = elPe
Homogeneous Equation
.dydv Logarithmic
y=vx and --:=r'rx- lna
dx dx a -e
x
a -e xlna
x
rX,A
)a dx =-*c
lna
GTNTRAT SOLUTION FOR z.d OBDIB DIFFfRENTIAL EQUATION

d'y . dv
I a::-l+b-'+crr=0
: Equation of the form dx' dx

-b+ - 4ac
Quadratics Formula X=
2a

1,. Real & different roots2 ! = Ae*'' + Be*'r

2. Real & equal roots y e*'(A+ Bx)
=
3. Complex roots ! = e"'(Acos px + Bsin px)