Page 30 - u4

P2: OSO/OVY
T1: OSO
QC: OSO/OVY
P1: OSO/OVY
GO01962-Smith-v1.cls
July 4, 2016
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
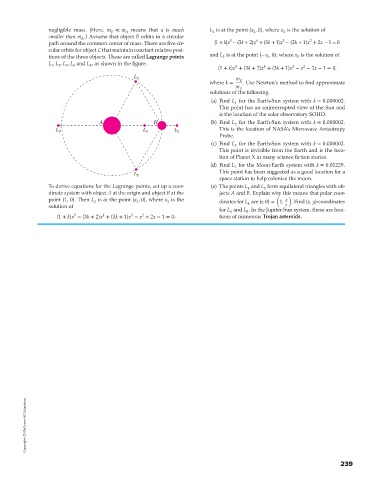
negligible mass. (Here, m ≪ m means that a is much 13:38 L is at the point (x , 0), where x is the solution of
A
2
2
B
2
smaller than m .) Assume that object B orbits in a circular
A
4
5
2
3
path around the common center of mass. There are five cir- (1 + k)x − (3k + 2)x + (3k + 1)x − (2k + 1)x + 2x − 1 = 0
cular orbits for object C that maintain constant relative posi-
tions of the three objects. These are called Lagrange points and L is at the point (−x , 0), where x is the solution of
3
3
3
L , L , L , L and L , as shown in the figure.
3
2
1
5
4
3
5
2
4
(1 + k)x + (3k + 2)x + (3k + 1)x − x − 2x − 1 = 0,
L 4 m
where k = B . Use Newton’s method to find approximate
m A
solutions of the following.
(a) Find L for the Earth-Sun system with k = 0.000002.
1
This point has an uninterrupted view of the Sun and
is the location of the solar observatory SOHO.
A B (b) Find L for the Earth-Sun system with k = 0.000002.
2
L 3 L 1 L 2 This is the location of NASA’s Microwave Anisotropy
Probe.
(c) Find L for the Earth-Sun system with k = 0.000002.
3
This point is invisible from the Earth and is the loca-
tion of Planet X in many science fiction stories.
(d) Find L for the Moon-Earth system with k = 0.01229.
1
This point has been suggested as a good location for a
L 5
space station to help colonize the moon.
To derive equations for the Lagrange points, set up a coor- (e) The points L and L form equilateral triangles with ob-
5
4
dinate system with object A at the origin and object B at the jects A and B. Explain why this means that polar coor-
(
point (1, 0). Then L is at the point (x , 0), where x is the dinates for L are (r, ) = 1, ) . Find (x, y)-coordinates
1
1
1
solution of 4 6
for L and L . In the Jupiter-Sun system, these are loca-
5
4
4
5
3
2
(1 + k)x − (3k + 2)x + (3k + 1)x − x + 2x − 1 = 0; tions of numerous Trojan asteroids.
4.2 INDETERMINATE FORMS AND l’HÔPITAL’S RULE
In this section, we reconsider the problem of computing limits. You have frequently
seen limits of the form
f(x)
lim ,
x→a g(x)
where lim f(x) = lim g(x) = 0 or where lim f(x) = lim g(x) =∞ (or −∞). Recall that from
x→a x→a x→a x→a
0
either of these forms ( or ∞ , called indeterminate forms), we cannot determine the
∞
0
value of the limit, or even whether the limit exists, without additional work. For in-
stance, note that
2
lim x − 1 = lim (x − 1)(x + 1) = lim x + 1 = 2 = 2,
x→1 x − 1 x→1 x − 1 x→1 1 1
lim x − 1 = lim x − 1 = lim 1 = 1
2
x→1 x − 1 x→1 (x − 1)(x + 1) x→1 x + 1 2
x − 1
and lim 2 x − 1 = lim (x − 1) 2 = lim 1 , which does not exist,
x→1 x − 1
x→1 x − 2x + 1
x→1
Copyright © McGraw-Hill Education even though all three limits initially have the form . The lesson here is that the ex-
0
0
0
pression
is mathematically meaningless. It indicates only that both the numerator
0
and denominator tend to zero and that we’ll need to dig deeper to find the value of the
limit or whether the limit even exists.
239