Page 33 - u4

P2: OSO/OVY
T1: OSO
QC: OSO/OVY
P1: OSO/OVY
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
GO01962-Smith-v1.cls
PROOF July 4, 2016 13:38
′
′
Here, we prove only the 0 0 case where f, f ,g and g are all continuous on all of (a, b) and
′
g (c) ≠ 0, while leaving the more intricate general 0 0 case for Appendix A. First, recall
the alternative form of the definition of derivative:
f(x) − f(c)
′
f (c) = lim .
x→c x − c
Working backward, we have by continuity that

f(x) − f(c) f(x) − f(c)
′
′
f (x) f (c) lim x − c x − c f(x) − f(c)
lim = = x→c = lim = lim .
′
′
x→c g (x) g (c) g(x) − g(c) x→c g(x) − g(c) x→c g(x) − g(c)
lim
x→c x − c x − c
Further, since f and g are continuous at x = c, we have that
f(c) = lim f(x) = 0 and g(c) = lim g(x) = 0.
x→c x→c
It now follows that

′
f (x) f(x) − f(c) f(x)
lim = lim = lim ,
′
x→c g (x) x→c g(x) − g(c) x→c g(x)
which is what we wanted.
We leave the proof for the ∞ case to more advanced texts.
∞

REMARK 2.1

f(x)
The conclusion of Theorem 2.1 also holds if lim is replaced with any of the
x→c g(x)
f(x) f(x) f(x) f(x)
limits lim , lim , lim or lim . (In each case, we must
−
x→c g(x) x→c g(x) x→∞ g(x) x→−∞ g(x)
+
make appropriate adjustments to the hypotheses.)

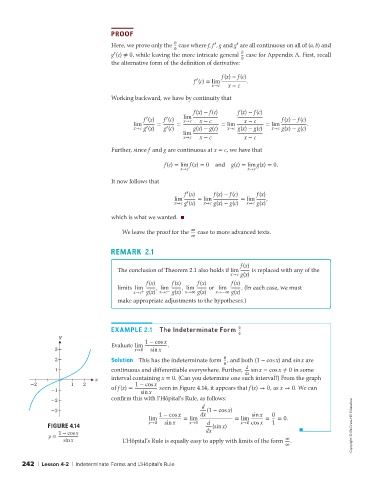
EXAMPLE 2.1 The Indeterminate Form 0
y 0
Evaluate lim 1 − cos x .
3 x→0 sin x
0
2 Solution This has the indeterminate form , and both (1 − cos x) and sin x are
0
d
1 continuous and differentiable everywhere. Further, dx sin x = cos x ≠ 0 in some
x interval containing x = 0. (Can you determine one such interval?) From the graph
-2 1 2 of f(x) = 1 − cos x seen in Figure 4.14, it appears that f(x) → 0, as x → 0. We can
-1 sin x
-2 confirm this with l’Ĥopital’s Rule, as follows:
d
-3 dx (1 − cos x)
lim 1 − cos x = lim = lim sin x = 0 = 0.
d
FIGURE 4.14 x→0 sin x x→0 dx (sin x) x→0 cos x 1 Copyright © McGraw-Hill Education
y = 1 − cos x
sin x L’Ĥopital’s Rule is equally easy to apply with limits of the form ∞ .
∞

242 | Lesson 4-2 | Indeterminate Forms and L’Hôpital’s Rule