Page 37 - u4

P2: OSO/OVY
QC: OSO/OVY
T1: OSO
P1: OSO/OVY
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
July 4, 2016
We now consider the limit 13:38
lim ln y = lim 1 ln x (∞ ⋅ 0)
+
x→1 + x→1 x − 1
ln x ( )
0
= lim
x→1 x − 1 0
+
d (ln x)
dx x −1
= lim = lim = 1. By l’Ĥopital’s Rule.
x→1 + d (x − 1) x→1 + 1
y dx
Be careful; we have found that lim ln y = 1, but this is not the original limit. We
1.0 x→1 +
want
0.9 lim y = lim e ln y = e ,
1
x→1 + x→1 +
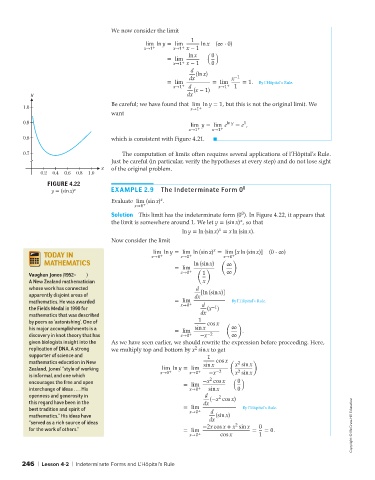
0.8 which is consistent with Figure 4.21.

0.7 The computation of limits often requires several applications of l’Ĥopital’s Rule.
Just be careful (in particular, verify the hypotheses at every step) and do not lose sight
x of the original problem.
0.2 0.4 0.6 0.8 1.0
FIGURE 4.22
y = (sin x) x EXAMPLE 2.9 The Indeterminate Form 0 0
x
Evaluate lim (sin x) .
x→0 +
0
Solution This limit has the indeterminate form (0 ). In Figure 4.22, it appears that
x
the limit is somewhere around 1. We let y = (sin x) , so that
x
ln y = ln (sin x) = x ln (sin x).
Now consider the limit
lim ln y = lim ln (sin x) = lim [x ln (sin x)] (0 ⋅ ∞)
TODAY IN x→0 + x→0 + x x→0 +
MATHEMATICS ln (sin x) ( ∞ )
= lim ( )
Vaughan Jones (1952– ) x→0 + 1 ∞
A New Zealand mathematician x
whose work has connected d
apparently disjoint areas of dx [ln (sin x)]
mathematics. He was awarded = lim + d By l’Ĥopital’s Rule.
−1
the Fields Medal in 1990 for x→0 (x )
mathematics that was described dx
by peers as ‘astonishing’. One of 1 cos x ( )
his major accomplishments is a = lim sin x ∞ .
discovery in knot theory that has x→0 + −x −2 ∞
given biologists insight into the As we have seen earlier, we should rewrite the expression before proceeding. Here,
2
replication of DNA. A strong we multiply top and bottom by x sin x to get
supporter of science and 1
mathematics education in New sin x cos x ( x sin x )
2
Zealand, Jones’ “style of working lim ln y = lim + −x −2 x sin x
2
+
is informal, and one which x→0 x→0
2
0
encourages the free and open = lim −x cos x ( )
interchange of ideas . . . . His x→0 + sin x 0
openness and generosity in d (−x cos x)
2
this regard have been in the dx
best tradition and spirit of = lim + d By l’Ĥopital’s Rule.
x→0
mathematics.” His ideas have dx (sin x)
“served as a rich source of ideas 2 Copyright © McGraw-Hill Education
for the work of others.” = lim −2x cos x + x sin x = 0 = 0.
x→0 + cos x 1

246 | Lesson 4-2 | Indeterminate Forms and L’Hôpital’s Rule