Page 40 - u4

T1: OSO
QC: OSO/OVY
P1: OSO/OVY
P2: OSO/OVY
GO01962-Smith-v1.cls
July 4, 2016
13:38
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
64. The size of an animal’s pupils expand and contract de-
APPLICATIONS
pending on the amount of light available. Let f(x) =
61. Previously ,we have briefly discussed the position of base- 160x −0.4 + 90 be the size in mm of the pupils at light inten-
ball thrown with the unusual knuckleball pitch. The left/ 8x −0.4 + 10
right position (in feet) of a ball thrown with spin rate sity x. Find lim f(x) and lim f(x), and argue that these rep-
x→∞
x→0 +
and a particular grip at time t seconds is f( ) = (2.5∕ )t − resent the largest and smallest possible sizes of the pupils,
2
(2.5∕4 ) sin 4 t. Treating t as a constant and as the vari- respectively.
able (change to x if you like), show that lim f( ) = 0 for
→0 65. The downward speed of a skydiver of mass m acted on by
any value of t. (Hint: Find a common denominator and use ( √ g )
l’Ĥopital’s Rule.) Conclude that a pitch with no spin does gravity and air drag is v = √ 40 mg tanh t . Find
not move left or right at all. 40 m
(a) lim v; (b) lim v; (c) lim v and state what each limit rep-
m→0 +
m→∞
t→∞
62. In this exercise, we look at a knuckleball thrown with a dif- resents in terms of the skydiver.
ferent grip than that of exercise 61. The left or right position
(in feet) of a ball thrown with spin rate and this new grip 66. The power of a reflecting telescope is proportional to
at time t seconds is f( ) = (2.5∕4 ) − (2.5∕4 ) sin (4 t + the surface area S of the parabolic reflector, with S =
2
2
∕2). Treating t as a constant and as the variable (change 8 2 [ ( t 2 ) 3∕2 ]
to x if you like), find lim f( ). Your answer should depend 3 c 2 + 1 − 1 for number c and d. Find lim S.
→0 16c c→∞
on t. By graphing this function of t, you can see the path
of the pitch (use a domain of 0 ≤ t ≤ 0.68). Describe this
pitch.
EXPLORATORY EXERCISES
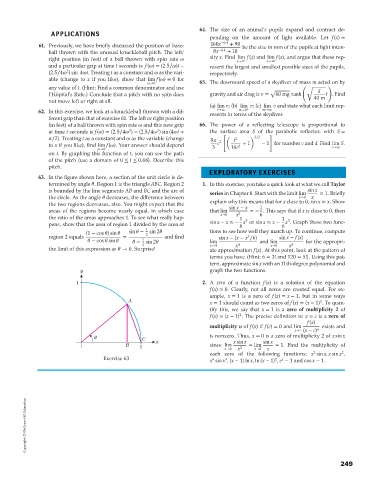
63. In the figure shown here, a section of the unit circle is de-
termined by angle . Region 1 is the triangle ABC. Region 2 1. In this exercise, you take a quick look at what we call Taylor
is bounded by the line segments AB and BC and the arc of series in Chapter 8. Start with the limit lim sin x = 1. Briefly
the circle. As the angle decreases, the difference between x→0 x
the two regions decreases, also. You might expect that the explain why this means that for x close to 0, sin x ≈ x. Show
1
areas of the regions become nearly equal, in which case that lim sin x − x =− . This says that if x is close to 0, then
3
the ratio of the areas approaches 1. To see what really hap- x→0 x 1 3 6 1 3
pens, show that the area of region 1 divided by the area of sin x − x ≈− x or sin x ≈ x − x . Graph these two func-
6
6
1
(1 − cos ) sin sin − sin 2 tions to see how well they match up. To continue, compute
region 2 equals = 2 and find sin x − (x − x ∕6) sin x − f(x)
3
1
− cos sin − sin 2 lim and lim for the appropri-
5
x
4
x
2
x→0
x→0
the limit of this expression as → 0. Surprise! ate approximation f(x). At this point, look at the pattern of
terms you have (Hint: 6 = 3! and 120 = 5!). Using this pat-
tern, approximate sin x with an 11th-degree polynomial and
y graph the two functions.
1 2. A zero of a function f(x) is a solution of the equation
f(x) = 0. Clearly, not all zeros are created equal. For ex-
ample, x = 1isazero of f(x) = x − 1, but in some ways
A x = 1 should count as two zeros of f(x) = (x − 1) . To quan-
2
tify this, we say that x = 1isa zero of multiplicity 2 of
f(x) = (x − 1) . The precise definition is: x = c is a zero of
2
f(x)
multiplicity n of f(x) if f(c) = 0 and lim exists and
x→c (x − c) n
θ C is nonzero. Thus, x = 0 is a zero of multiplicity 2 of x sin x
sin x
x sin x
B 1 x since lim x 2 = lim x = 1. Find the multiplicity of
x→0
x→0
2
2
each zero of the following functions: x sin x, x sin x ,
Exercise 63 4 3 2 x
x sin x , (x − 1) ln x, ln (x − 1) , e − 1 and cos x − 1.
4.3 MAXIMUM AND MINIMUM VALUES
Copyright © McGraw-Hill Education To remain competitive, businesses must regularly evaluate how to minimize waste and
maximize the return on their investment. In this section, we consider the problem of
finding maximum and minimum values of functions. In section 3.7, we examine how
to apply these notions to problems of an applied nature.
We begin by giving careful mathematical definitions of some familiar terms.
249